Last month, I discussed a fresh paper by Kyriakos Papadodimas about the creation of objects inside a black hole using operators that exist outside, if I put it in catchy words.
Since that time, I was returning to my old tempting ideas that the black hole complementarity – the dependence relating the black hole interior and the black hole exterior – could be much simpler than we thought, given by some formula, and that this formula could be rationally justifiable or provable by a rock-solid, physically understandable, nearly rigorous argument.
This viewpoint was energized yesterday when John Preskill tweeted:
Recall that Beni Yoshida is a Japanese quantum gravity expert who explained the relationship of quantum gravity and error-correcting codes in his 2015 TRF blog post.
Now, Preskill referred to a Quantum Error Correction conference that is ending in Maryland today. If you find the talk or a relevant paper by Yoshida, I will be very interested.
For years, I thought that "with some clarifications", the black hole interior operators could be some analytic continuations of those outside. We know that this can't be quite true in the Rindler space. If you draw the Minkowski space and identify some coordinates \(r^2=x^\mu x_\mu\) and \({\rm tanh}\,\eta=t/z\) and wedges, you could also say that e.g. the wedge with \(z\lt 0\) is an analytic continuation of the wedge with \(z \gt 0\) to some complex values of the rapidity \(\eta\).
That sounds great but the devil is in the details. We know that the operators in the two wedges should be completely independent of each other and commuting with each other – because they are spacelike separated. A related, perhaps complementary, problem is that the operators shouldn't really be expected to be very smooth, analytic functions of the coordinate \(\eta\), or other coordinates.
I believe that the black hole interior must have some very specific property that allows to identify these two problems. Exactly when you consider the black hole interior and not the empty Rindler space, some kind of a simple continuation similar to one proposed above could become right and justifiable. And maybe it's not an analytic continuation, after all.
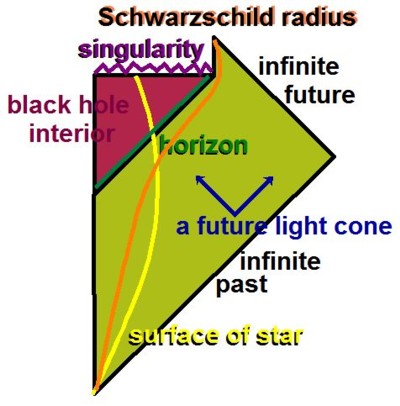
Instead, there could exist some functional formula relating the operators in the black hole spacetime that follows from the assumptions about the black hole spacetime and that relates the operators inside and outside. What could it be? Look at the Penrose diagram of an evaporating neutral black hole above.
There is some singularity and I believe that you shouldn't really assume much about it. The spacetime fields cease to be applicable somewhere near the singularity but the precise place or moment when it happens is impossible to define. On the other hand, look at the corner at the right endpoint of the teeth-like singularity. It's the event where the already nearly evaporated black hole finally disappears. And from that event to the top, the empty Minkowski spacetime continues. Let me call this point \(Y\) because it appears near "y" of the word "singularity" on the picture. It's the intersection of the singularity with the post-black-hole Minkowski spacetime – which also happens to be the latest point of the event horizon.
Is this causal diagram unavoidable for a given evolution of the black hole spacetime? Should it be generalized? And whatever are the answers and/or the generalization, doesn't the condition that the future spacetime looks like "this", or has some of these features, represent some condition constraining the field operators outside and inside the black hole?
Recall that I have said that in the Rindler space – or the Minkowski space – operators are not really analytic functions of the spacetime coordinates. If there is some relationship between the interior and exterior operator that can be derived in the true black hole spacetimes but not in the empty Minkowski or Rindler space, it should be because the black hole spacetime makes the fields somewhere, probably near the horizon or around its "last point" discussed above, more analytic than it is in the case of the empty spacetime. Or there should be some condition on the operators saying that "the evolution of the fields is compatible with a peaceful disappearance of the black hole including the singularity".
In particular, I think that the singularity could generically remain "very singular" even if you look at the right end of the singular teeth in the causal diagram above. I am not quite sure about this point – the black hole is getting smaller as it evaporates, and so does the "absolute magnitude" of the singular terms in the fields, but maybe the relative size of the singular and non-singular terms is what matters and must go to zero. But near the point \(Y\), if this guess is right, the singularity is obliged to "desingularize itself", to allow the existence of the smooth spacetime after the black hole has evaporated, and this conditions may nontrivial relate the field operators at earlier times, too.
The condition on the fields at some slice could say that "the last moment \(Y\) of the black hole that you get by evolving the spacetime to the future has some potentially singular quantities equal to zero". And this is the condition that could say that in some sense, the interior fields are some continuations of the exterior fields, after all.
When you integrate out the fields in the left wedge of the Rindler space, you get the Unruh thermal radiation in the right wedge. The integration out is equivalent to the ignorance about some phases of the fields and/or creation and annihilation operators. It could be exactly these phases that are determined by the condition of the "smooth end of the black hole at point \(Y\)" that I have discussed above.

Since that time, I was returning to my old tempting ideas that the black hole complementarity – the dependence relating the black hole interior and the black hole exterior – could be much simpler than we thought, given by some formula, and that this formula could be rationally justifiable or provable by a rock-solid, physically understandable, nearly rigorous argument.
This viewpoint was energized yesterday when John Preskill tweeted:
Beni Yoshida explains that decoding the Hawking radiation emitted by a black hole is easier than we thought. #QEC17
— John Preskill (@preskill) September 14, 2017
Recall that Beni Yoshida is a Japanese quantum gravity expert who explained the relationship of quantum gravity and error-correcting codes in his 2015 TRF blog post.
Now, Preskill referred to a Quantum Error Correction conference that is ending in Maryland today. If you find the talk or a relevant paper by Yoshida, I will be very interested.
For years, I thought that "with some clarifications", the black hole interior operators could be some analytic continuations of those outside. We know that this can't be quite true in the Rindler space. If you draw the Minkowski space and identify some coordinates \(r^2=x^\mu x_\mu\) and \({\rm tanh}\,\eta=t/z\) and wedges, you could also say that e.g. the wedge with \(z\lt 0\) is an analytic continuation of the wedge with \(z \gt 0\) to some complex values of the rapidity \(\eta\).
That sounds great but the devil is in the details. We know that the operators in the two wedges should be completely independent of each other and commuting with each other – because they are spacelike separated. A related, perhaps complementary, problem is that the operators shouldn't really be expected to be very smooth, analytic functions of the coordinate \(\eta\), or other coordinates.
I believe that the black hole interior must have some very specific property that allows to identify these two problems. Exactly when you consider the black hole interior and not the empty Rindler space, some kind of a simple continuation similar to one proposed above could become right and justifiable. And maybe it's not an analytic continuation, after all.
Instead, there could exist some functional formula relating the operators in the black hole spacetime that follows from the assumptions about the black hole spacetime and that relates the operators inside and outside. What could it be? Look at the Penrose diagram of an evaporating neutral black hole above.
There is some singularity and I believe that you shouldn't really assume much about it. The spacetime fields cease to be applicable somewhere near the singularity but the precise place or moment when it happens is impossible to define. On the other hand, look at the corner at the right endpoint of the teeth-like singularity. It's the event where the already nearly evaporated black hole finally disappears. And from that event to the top, the empty Minkowski spacetime continues. Let me call this point \(Y\) because it appears near "y" of the word "singularity" on the picture. It's the intersection of the singularity with the post-black-hole Minkowski spacetime – which also happens to be the latest point of the event horizon.
Is this causal diagram unavoidable for a given evolution of the black hole spacetime? Should it be generalized? And whatever are the answers and/or the generalization, doesn't the condition that the future spacetime looks like "this", or has some of these features, represent some condition constraining the field operators outside and inside the black hole?
Recall that I have said that in the Rindler space – or the Minkowski space – operators are not really analytic functions of the spacetime coordinates. If there is some relationship between the interior and exterior operator that can be derived in the true black hole spacetimes but not in the empty Minkowski or Rindler space, it should be because the black hole spacetime makes the fields somewhere, probably near the horizon or around its "last point" discussed above, more analytic than it is in the case of the empty spacetime. Or there should be some condition on the operators saying that "the evolution of the fields is compatible with a peaceful disappearance of the black hole including the singularity".
In particular, I think that the singularity could generically remain "very singular" even if you look at the right end of the singular teeth in the causal diagram above. I am not quite sure about this point – the black hole is getting smaller as it evaporates, and so does the "absolute magnitude" of the singular terms in the fields, but maybe the relative size of the singular and non-singular terms is what matters and must go to zero. But near the point \(Y\), if this guess is right, the singularity is obliged to "desingularize itself", to allow the existence of the smooth spacetime after the black hole has evaporated, and this conditions may nontrivial relate the field operators at earlier times, too.
The condition on the fields at some slice could say that "the last moment \(Y\) of the black hole that you get by evolving the spacetime to the future has some potentially singular quantities equal to zero". And this is the condition that could say that in some sense, the interior fields are some continuations of the exterior fields, after all.
When you integrate out the fields in the left wedge of the Rindler space, you get the Unruh thermal radiation in the right wedge. The integration out is equivalent to the ignorance about some phases of the fields and/or creation and annihilation operators. It could be exactly these phases that are determined by the condition of the "smooth end of the black hole at point \(Y\)" that I have discussed above.
Can decoding of Hawking radiation be easy?
 Reviewed by DAL
on
September 14, 2017
Rating:
Reviewed by DAL
on
September 14, 2017
Rating:




No comments: