Wrong symmetries, misleading number of vertices
Eclectikus told us about this 3-week old video
proposing a new notation for the exponentiation based on triangles. A similar issue was discussed five years ago at Mathematics Stack Exchange. Well, it's amusing and sometimes useful to invent alternative notations and I had to do it many times in the past (especially whenever I needed to deal with lots of algebra and hundreds of terms of some kind). But I just disagree with this particular one – and so does Eclectikus.
I think that this topic is a good example of the good and bad thinking and relevant facts in many proposed reforms of this kind. Also, I remember in detail how my understanding of the powers was evolving since the age of 5, what was right about it and what was not. So let's start.
The principal justification for the triangle notation of powers is the dissatisfaction about the fact that there are three very different ways how to write down a basically equivalent fact:\[
\Large
\eq{
2^3 &= 8 \\
\sqrt[3]{8} &= 2 \\
\log_2(8) &= 3
}
\] If the statements are equivalent, shouldn't the ways how we write them be similar as well?
I think that the answer is a resounding No. The reason is that we're using these symbols not just to write the particular statement of the form \(a^b=c\). We're using these three symbols to write the actual three functions of two variables – functions that may be used as parts of more complicated, composite functions and expressions and equations. And they're very different functions.
The video suggests to write all three functions using an equilateral triangle with some symbols sometimes written next to the 2 vertices:\[
\begin{align}
x^y &={} \stackrel{y}{_x\triangle_{\phantom{z}}}&&\text{$x$ to the $y$}\\
\sqrt[y]{z} &={} \stackrel{y}{_\phantom{x}\triangle_{z}}&&\text{$y$th root of $z$}\\
\log_x(z)&={} \stackrel{}{_x\triangle_{z}}&&\text{log base $x$ of $z$}\\
\end{align}
\] The lines with the triangles are pretty, similar, and symmetric. But the question you should ask is: Should they be? The correct answer is No (at least to 2 of the 3 adjectives). They shouldn't be similar or symmetric because the functions are qualitatively different and a good system of notation makes their qualitative difference as obvious as possible.
In particular, the exponent \(3\) plays a very different role in \(2^3=8\) than the other two numbers \(2,8\). The triangle notation – especially because it looks like an equilateral triangle – indicates that there is some form of an \(S_3\) symmetry between the three numbers. But there's no such symmetry. The three numbers play very different roles. And that's the asymmetry that a faithful notation should make obvious.
I suspect that lots of people who support such triangles just don't "feel" how internally different the exponentiation and logarithms are. For them, \(\exp\) and \(\ln\) are just two buttons on a calculator that may be pressed and that change a number to another. But what's happening inside is a black box for them. They aren't really familiar with the huge differences in the identities involving \(\exp\) and \(\ln\), respectively, and so on.
The base and the resulting power play different roles, too. With the triangle notation, there would be constant confusions and mistakes caused by a permutation of two or three of the vertices. To confuse the base and the exponent is a serious mistake, one that completely changes the character of the expression. The very idea that you "should" have a similar symbol for the exponential and the logarithm is analogous to the claim that the verbs "giving a birth" and "murdering" should have similar words because they're inverse and therefore analogous to one another, too.
Why don't we say "to durmer" instead of "to give a birth" in order to make the symmetry with "to murder" more explicit? Well, we don't and I think that rational people know that it would be counterproductive, not great, to have similar or symmetric words for births and murders. They're just different things – also acts that require a very different kind of work (just like \(\exp\) and \(\ln\) do), despite the results' being opposite to each other – and it's very important to distinguish them. In the same way, we could say "positive and sopitive" instead of "positive and negative". Again, these two words would get confused often. Creators of usable languages have deliberately used different words for these "inverse" operations because the difference – the need to distinguish them – is so important.
I think that the desire to make symbols symmetric even though the underlying objects obviously don't have the symmetry is one of the signs of the widespread fanatical egalitarianism and leftism. A huge part of the leftist contamination of the contemporary Western society may be described as examples of the aggressively spread lie that things and people are the same even though they self-evidently and importantly aren't. And some people want to spread this lie to the world of bases, exponents, and powers, too.

Even the Byzantine missionaries sent to Great Moravia (Czechoslovakia) in 863 were obsessed with counterproductive symmetric symbols. Saints Cyril and Methodius developed a new glagolitic alphabet to faithfully reproduce the Slavic sounds. Using this alphabet, the Bible was translated to the Old Church Slavonic – the first regular people's tongue legitimized as a religious language after the "classic" religious languages such as Hebrew, Greek, and Latin. Fair enough. But you may see that an unnaturally high fraction of the symbols have a left-right or up-down mirror symmetry. They're really too redundant and more complex (and therefore more time-consuming) than they could be (an example of a text). Couldn't they have used the Greek alphabet with some diacritics, for example? Within a century, this script disappeared from Czechia, anyway, along with the Eastern Christianity. They were replaced by the Catholic belief and the Latin alphabet. And it was soon realized that the Latin alphabet is just fine for the Slavic sounds, with some extra two-letter symbols. Diacritics were introduced in the early 15th century. Meanwhile, in the 9th century, Eastern Slavs did start to write in Cyrillic script which was a variation of the Greek alphabet. Some Cyrillic letters were also too symmetric and too redundant but most of them went extinct and at the end, the Greek, Latin, and Cyrillic scripts look comparably simple (complex) and comparably (a)symmetric today.
But the symmetry vs asymmetry dispute isn't the only problem of the triangle notation. Another problem is the very suggestion that the operation has fundamentally three players. Why?
When I was 5, I learned about exponentiation for the first time and it looked great. At that moment, I thought that there were five operations. I also learned about complex numbers and sometimes when I was a first-grader, I was obsessed by the desire to know how much is \(a^b\) for any \(a,b\in\CC\). I was already convinced that the complex numbers allow you to calculate anything, that the annoying "E" result that my calculator sometimes returned doesn't happen in the paradise-like world of complex numbers, and so on.
(I also established a math-teaching club when I was a first grader, working hard to share the joy of \(\pi\), factorials, powers, and complex numbers with my friends. We mostly did non-mathematical things, however, like physically facing a gang of gypsies. Also, my friend Ota K. taught me that a woman in the street had had sex; and that when people want to have children, they have to use a condom.)
With hindsight, it looks strange that I was so obsessed with such a technicality. But the reasons are similar to the reasons why I ever found it important to memorize the first 30 digits of \(\pi\) (three years later, I memorized the following 70 digits only because of fun – I saw that it is a way to impress some people in a way that looked easy to me; but I already knew it was pretty much pointless): I simply had oversimplified and narrow-minded ideas about "how much important mathematics is out there and what is actually important". So learning an important number such as \(\pi\) or the methods to calculate the power \(a^b\) for any two allowed (complex) numbers looked like a "big chunk of mathematics", to say the least. It was rubbish, of course, but I couldn't have quite known it was rubbish before I learned much more about mathematics and physics etc.
But why I am saying these things about the childhood? Some two years later, I did learn how to (numerically) compute \(a^b\) for specific numbers \(a,b\in\CC\). As you know, the exponentiation may be reduced to the standard exponential:\[
\Large
a^b = \exp(b\cdot \ln(a))
\] The funny thing is that you don't need a general complex function of two variables, the general exponentiation. One function of one variable (the exponential) and its inverse (the natural logarithm) are enough!
The more experienced physicist (or calculus-based mathematician) one becomes (and I was becoming), the more he or (less likely) she realizes that the natural "systemic" way to talk about the general powers is the exponential with the base \(e=2.718\dots\). Of course, one has to understand why the base \(e\) is more natural, even relatively to widespread bases such as \(2\) and \(10\). The exponential \(\exp(x)=e^x\) is the only function of \(x\) among functions \(b^x\) whose derivative is exactly the same function, for example. Equivalently, the derivative of \(\ln(x)\) is \(1/x\) and this simplest result also works for the base \(b=e\) only.
Consequently, the Taylor expansions of functions \(e^x\) and \(\ln(x)\) are simpler than the expansions of other general powers, and so on. I do think that the "example with the interest rates" was my first encounter with the importance of \(e\) as a base. Take one Czechoslovak crown (to return the currencies of the 1980s). After one year and 100% yields, you turn CSK 1 to CSK 2. If you get 50% twice a year, you will own CSK 1.5 x 1.5 = CSK 2.25 after the first year. Try to get 10% ten times. You get CSK 1.110 = CSK 2.59 etc. If you get the smaller interest more frequently, in the limit of the high frequency, you will only end up with a finite amount of money, namely CSK 2.718...
OK, the base \(b=e\) is the most natural base among those that may produce "all" numbers at all – note that the base \(b=1\) is no good for logarithms because all the logarithms would be ill-defined. Once you realize that it's really just the simple functions of 1 variable that are fundamental, you may write\[
\eq{
x^y &= \exp(y\ln (x))\\
\sqrt[u]{v} &= \exp(\ln(v)/u)\\
\log_c(d) &= \frac{\ln d}{\ln c}.
}
\] Nice. You see that the general results aren't quite well-defined for complex values of the argument because the complex function \(\ln(z)\) is only defined up to an arbitrary additive shift \(+2\pi i k\) where \(k\in\ZZ\) is any integer. So the general power (or root or logarithm) involving two (finite, nonzero) complex numbers that I wanted to be able to calculate was calculable but the result wasn't unique. That was a kind of a resolution I didn't quite expect but this very fact was also an important lesson: Questions sometimes have answers you don't expect, answers "in the middle", answers with disclaimers and exceptions, and so on.
Incidentally, the realization that \(e^x\) and \(\ln(x)\) are the only functions that you really need has affected me, too. I quickly realized that even multiplication and division may be eliminated from the list of basic operations because \[
\eq{
u\cdot v &= \exp(\ln u + \ln v)\\
\frac{u}{v} &= \exp(\ln u - \ln v)
}
\] which means that the addition, subtraction, exponential, and natural logarithm are the only operations you need. Just to be sure, you may kill the subtraction as well because\[
u - v = u + \exp(\ln v + \pi i).
\] Well, assuming that I am allowed to write down complex constants such as \(\pi i\). I don't want to make it too ludicrous. Subtraction is so elementary and widespread that you don't want to replace it by complicated functions involving exponentials and logarithms. In practice, I haven't even "used" the way to rewrite the general product in terms of the exponential, logarithm, and addition. It's an identity you sometimes need but as a tool to deal with general products, it's just a curiosity that you may survive without a standalone product operation. It's often nice to reduce the number of basic operations and concepts but if you're too fanatical about it, you may do more harm than good, too.
But the point I want to make is that not only the triangle notation is a problematic way to represent particular elementary objects – an unjustifiably symmetric representation of a goal. What's worse: the goal itself is misguided. The very fact that it's a triangle that looks "fundamental" suggests that some complicated function of two variables – i.e. identities with 3 players (including the result) – has to be added to mathematics.
As the kids should learn a bit later, perhaps by the end of the high school, this is not really the case. The fundamental operations you need to calculate the general powers and general logarithms are functions of one variable only – you would need "diangles" for those, not "triangles". The triangle looks like an irreducible relationship with 3 players but the underlying entity is not irreducible. And in physics, \(\exp(x)\) and \(\ln(y)\) appears far more frequently than the power \(b^x\) and logarithm \(\ln_b(y)\) with a more general base \(b\). Also, \(\sqrt{x}\) is the base-two (square) root and appears frequently enough so that it's good to have a special, simple enough symbol, instead of a triangle with the number \(2\) written next to one of the vertices that I can't reliably pinpoint even if I think for 5 seconds.
Changes of notation may be costly because the transition may cause lots of problems. But sometimes they may make sense. However, I am afraid that with the current balance of power, especially in the Western education systems, such reforms are being pushed by people who don't think about (and don't discuss) the consequences as described in this blog post (just a very specific example) and, in many cases, they are completely ignorant of some very basic concepts and principles (e.g. the general logarithm of a complex number etc.) which means that they can't even think or discuss these important issues.
That's why a vast majority of such proposed changes are unavoidably counterproductive. Mathematicians have used the same notation for powers and logarithms for a few centuries and if it had some obvious enough and easy-to-fix problems or redundancies, they would have already fixed those problems. Most of the people who want to make the fixes are usually missing something important so a majority of the proposed changes would make the notation (or more important things) worse, not better.
Eclectikus told us about this 3-week old video
proposing a new notation for the exponentiation based on triangles. A similar issue was discussed five years ago at Mathematics Stack Exchange. Well, it's amusing and sometimes useful to invent alternative notations and I had to do it many times in the past (especially whenever I needed to deal with lots of algebra and hundreds of terms of some kind). But I just disagree with this particular one – and so does Eclectikus.
I think that this topic is a good example of the good and bad thinking and relevant facts in many proposed reforms of this kind. Also, I remember in detail how my understanding of the powers was evolving since the age of 5, what was right about it and what was not. So let's start.
The principal justification for the triangle notation of powers is the dissatisfaction about the fact that there are three very different ways how to write down a basically equivalent fact:\[
\Large
\eq{
2^3 &= 8 \\
\sqrt[3]{8} &= 2 \\
\log_2(8) &= 3
}
\] If the statements are equivalent, shouldn't the ways how we write them be similar as well?
I think that the answer is a resounding No. The reason is that we're using these symbols not just to write the particular statement of the form \(a^b=c\). We're using these three symbols to write the actual three functions of two variables – functions that may be used as parts of more complicated, composite functions and expressions and equations. And they're very different functions.
The video suggests to write all three functions using an equilateral triangle with some symbols sometimes written next to the 2 vertices:\[
\begin{align}
x^y &={} \stackrel{y}{_x\triangle_{\phantom{z}}}&&\text{$x$ to the $y$}\\
\sqrt[y]{z} &={} \stackrel{y}{_\phantom{x}\triangle_{z}}&&\text{$y$th root of $z$}\\
\log_x(z)&={} \stackrel{}{_x\triangle_{z}}&&\text{log base $x$ of $z$}\\
\end{align}
\] The lines with the triangles are pretty, similar, and symmetric. But the question you should ask is: Should they be? The correct answer is No (at least to 2 of the 3 adjectives). They shouldn't be similar or symmetric because the functions are qualitatively different and a good system of notation makes their qualitative difference as obvious as possible.
In particular, the exponent \(3\) plays a very different role in \(2^3=8\) than the other two numbers \(2,8\). The triangle notation – especially because it looks like an equilateral triangle – indicates that there is some form of an \(S_3\) symmetry between the three numbers. But there's no such symmetry. The three numbers play very different roles. And that's the asymmetry that a faithful notation should make obvious.
I suspect that lots of people who support such triangles just don't "feel" how internally different the exponentiation and logarithms are. For them, \(\exp\) and \(\ln\) are just two buttons on a calculator that may be pressed and that change a number to another. But what's happening inside is a black box for them. They aren't really familiar with the huge differences in the identities involving \(\exp\) and \(\ln\), respectively, and so on.
The base and the resulting power play different roles, too. With the triangle notation, there would be constant confusions and mistakes caused by a permutation of two or three of the vertices. To confuse the base and the exponent is a serious mistake, one that completely changes the character of the expression. The very idea that you "should" have a similar symbol for the exponential and the logarithm is analogous to the claim that the verbs "giving a birth" and "murdering" should have similar words because they're inverse and therefore analogous to one another, too.
Why don't we say "to durmer" instead of "to give a birth" in order to make the symmetry with "to murder" more explicit? Well, we don't and I think that rational people know that it would be counterproductive, not great, to have similar or symmetric words for births and murders. They're just different things – also acts that require a very different kind of work (just like \(\exp\) and \(\ln\) do), despite the results' being opposite to each other – and it's very important to distinguish them. In the same way, we could say "positive and sopitive" instead of "positive and negative". Again, these two words would get confused often. Creators of usable languages have deliberately used different words for these "inverse" operations because the difference – the need to distinguish them – is so important.
I think that the desire to make symbols symmetric even though the underlying objects obviously don't have the symmetry is one of the signs of the widespread fanatical egalitarianism and leftism. A huge part of the leftist contamination of the contemporary Western society may be described as examples of the aggressively spread lie that things and people are the same even though they self-evidently and importantly aren't. And some people want to spread this lie to the world of bases, exponents, and powers, too.
Even the Byzantine missionaries sent to Great Moravia (Czechoslovakia) in 863 were obsessed with counterproductive symmetric symbols. Saints Cyril and Methodius developed a new glagolitic alphabet to faithfully reproduce the Slavic sounds. Using this alphabet, the Bible was translated to the Old Church Slavonic – the first regular people's tongue legitimized as a religious language after the "classic" religious languages such as Hebrew, Greek, and Latin. Fair enough. But you may see that an unnaturally high fraction of the symbols have a left-right or up-down mirror symmetry. They're really too redundant and more complex (and therefore more time-consuming) than they could be (an example of a text). Couldn't they have used the Greek alphabet with some diacritics, for example? Within a century, this script disappeared from Czechia, anyway, along with the Eastern Christianity. They were replaced by the Catholic belief and the Latin alphabet. And it was soon realized that the Latin alphabet is just fine for the Slavic sounds, with some extra two-letter symbols. Diacritics were introduced in the early 15th century. Meanwhile, in the 9th century, Eastern Slavs did start to write in Cyrillic script which was a variation of the Greek alphabet. Some Cyrillic letters were also too symmetric and too redundant but most of them went extinct and at the end, the Greek, Latin, and Cyrillic scripts look comparably simple (complex) and comparably (a)symmetric today.
But the symmetry vs asymmetry dispute isn't the only problem of the triangle notation. Another problem is the very suggestion that the operation has fundamentally three players. Why?
When I was 5, I learned about exponentiation for the first time and it looked great. At that moment, I thought that there were five operations. I also learned about complex numbers and sometimes when I was a first-grader, I was obsessed by the desire to know how much is \(a^b\) for any \(a,b\in\CC\). I was already convinced that the complex numbers allow you to calculate anything, that the annoying "E" result that my calculator sometimes returned doesn't happen in the paradise-like world of complex numbers, and so on.
(I also established a math-teaching club when I was a first grader, working hard to share the joy of \(\pi\), factorials, powers, and complex numbers with my friends. We mostly did non-mathematical things, however, like physically facing a gang of gypsies. Also, my friend Ota K. taught me that a woman in the street had had sex; and that when people want to have children, they have to use a condom.)
With hindsight, it looks strange that I was so obsessed with such a technicality. But the reasons are similar to the reasons why I ever found it important to memorize the first 30 digits of \(\pi\) (three years later, I memorized the following 70 digits only because of fun – I saw that it is a way to impress some people in a way that looked easy to me; but I already knew it was pretty much pointless): I simply had oversimplified and narrow-minded ideas about "how much important mathematics is out there and what is actually important". So learning an important number such as \(\pi\) or the methods to calculate the power \(a^b\) for any two allowed (complex) numbers looked like a "big chunk of mathematics", to say the least. It was rubbish, of course, but I couldn't have quite known it was rubbish before I learned much more about mathematics and physics etc.
But why I am saying these things about the childhood? Some two years later, I did learn how to (numerically) compute \(a^b\) for specific numbers \(a,b\in\CC\). As you know, the exponentiation may be reduced to the standard exponential:\[
\Large
a^b = \exp(b\cdot \ln(a))
\] The funny thing is that you don't need a general complex function of two variables, the general exponentiation. One function of one variable (the exponential) and its inverse (the natural logarithm) are enough!
The more experienced physicist (or calculus-based mathematician) one becomes (and I was becoming), the more he or (less likely) she realizes that the natural "systemic" way to talk about the general powers is the exponential with the base \(e=2.718\dots\). Of course, one has to understand why the base \(e\) is more natural, even relatively to widespread bases such as \(2\) and \(10\). The exponential \(\exp(x)=e^x\) is the only function of \(x\) among functions \(b^x\) whose derivative is exactly the same function, for example. Equivalently, the derivative of \(\ln(x)\) is \(1/x\) and this simplest result also works for the base \(b=e\) only.
Consequently, the Taylor expansions of functions \(e^x\) and \(\ln(x)\) are simpler than the expansions of other general powers, and so on. I do think that the "example with the interest rates" was my first encounter with the importance of \(e\) as a base. Take one Czechoslovak crown (to return the currencies of the 1980s). After one year and 100% yields, you turn CSK 1 to CSK 2. If you get 50% twice a year, you will own CSK 1.5 x 1.5 = CSK 2.25 after the first year. Try to get 10% ten times. You get CSK 1.110 = CSK 2.59 etc. If you get the smaller interest more frequently, in the limit of the high frequency, you will only end up with a finite amount of money, namely CSK 2.718...
OK, the base \(b=e\) is the most natural base among those that may produce "all" numbers at all – note that the base \(b=1\) is no good for logarithms because all the logarithms would be ill-defined. Once you realize that it's really just the simple functions of 1 variable that are fundamental, you may write\[
\eq{
x^y &= \exp(y\ln (x))\\
\sqrt[u]{v} &= \exp(\ln(v)/u)\\
\log_c(d) &= \frac{\ln d}{\ln c}.
}
\] Nice. You see that the general results aren't quite well-defined for complex values of the argument because the complex function \(\ln(z)\) is only defined up to an arbitrary additive shift \(+2\pi i k\) where \(k\in\ZZ\) is any integer. So the general power (or root or logarithm) involving two (finite, nonzero) complex numbers that I wanted to be able to calculate was calculable but the result wasn't unique. That was a kind of a resolution I didn't quite expect but this very fact was also an important lesson: Questions sometimes have answers you don't expect, answers "in the middle", answers with disclaimers and exceptions, and so on.
Incidentally, the realization that \(e^x\) and \(\ln(x)\) are the only functions that you really need has affected me, too. I quickly realized that even multiplication and division may be eliminated from the list of basic operations because \[
\eq{
u\cdot v &= \exp(\ln u + \ln v)\\
\frac{u}{v} &= \exp(\ln u - \ln v)
}
\] which means that the addition, subtraction, exponential, and natural logarithm are the only operations you need. Just to be sure, you may kill the subtraction as well because\[
u - v = u + \exp(\ln v + \pi i).
\] Well, assuming that I am allowed to write down complex constants such as \(\pi i\). I don't want to make it too ludicrous. Subtraction is so elementary and widespread that you don't want to replace it by complicated functions involving exponentials and logarithms. In practice, I haven't even "used" the way to rewrite the general product in terms of the exponential, logarithm, and addition. It's an identity you sometimes need but as a tool to deal with general products, it's just a curiosity that you may survive without a standalone product operation. It's often nice to reduce the number of basic operations and concepts but if you're too fanatical about it, you may do more harm than good, too.
But the point I want to make is that not only the triangle notation is a problematic way to represent particular elementary objects – an unjustifiably symmetric representation of a goal. What's worse: the goal itself is misguided. The very fact that it's a triangle that looks "fundamental" suggests that some complicated function of two variables – i.e. identities with 3 players (including the result) – has to be added to mathematics.
As the kids should learn a bit later, perhaps by the end of the high school, this is not really the case. The fundamental operations you need to calculate the general powers and general logarithms are functions of one variable only – you would need "diangles" for those, not "triangles". The triangle looks like an irreducible relationship with 3 players but the underlying entity is not irreducible. And in physics, \(\exp(x)\) and \(\ln(y)\) appears far more frequently than the power \(b^x\) and logarithm \(\ln_b(y)\) with a more general base \(b\). Also, \(\sqrt{x}\) is the base-two (square) root and appears frequently enough so that it's good to have a special, simple enough symbol, instead of a triangle with the number \(2\) written next to one of the vertices that I can't reliably pinpoint even if I think for 5 seconds.
Changes of notation may be costly because the transition may cause lots of problems. But sometimes they may make sense. However, I am afraid that with the current balance of power, especially in the Western education systems, such reforms are being pushed by people who don't think about (and don't discuss) the consequences as described in this blog post (just a very specific example) and, in many cases, they are completely ignorant of some very basic concepts and principles (e.g. the general logarithm of a complex number etc.) which means that they can't even think or discuss these important issues.
That's why a vast majority of such proposed changes are unavoidably counterproductive. Mathematicians have used the same notation for powers and logarithms for a few centuries and if it had some obvious enough and easy-to-fix problems or redundancies, they would have already fixed those problems. Most of the people who want to make the fixes are usually missing something important so a majority of the proposed changes would make the notation (or more important things) worse, not better.
Why the triangle notation for powers is misguided
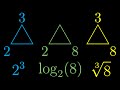 Reviewed by MCH
on
July 18, 2016
Rating:
Reviewed by MCH
on
July 18, 2016
Rating:
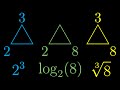 Reviewed by MCH
on
July 18, 2016
Rating:
Reviewed by MCH
on
July 18, 2016
Rating:



No comments: