The music analogy is much more accurate than most people want to believe
Tetragraviton is a postdoc at the Perimeter Institute who has written several papers on multiloop amplitudes in gauge theory. Even though none of these papers depends on string theory in any tangible way, I've thought that he's a guy close enough to string theory who could potentially work on it which is why I was surprised by his blog post a week ago,
Needless to say, the claim that (in weakly coupled string theory) different particle species are vibration modes of a string isn't just some fairy-tale used by Brian Greene. It's a translation of an actual defining fact of string theory into plain English. Brian Greene has in no way a monopoly over such a thing. Pretty much everyone else who has talked about string theory agrees that this is the right summary of string theory's ingenious description of the diversity of particle species.
Clearly, you may add people like Michio Kaku:
It's always amazing to see how many people like to pick an important truth, completely negate it, and claim that the result is a very important truth. It looks like they want to prove Niels Bohr's famous quote
OK, so how did Tetragraviton argue that particles aren't vibrations?
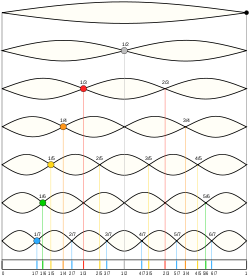
We were shown the higher harmonics on a string with a claim that this is not how string theory produces the list of particle species. Except that it is a totally valid sketch of how string theory does it.
In a flat spacetime background, a single string really has possible higher harmonics \(\alpha^\mu_{\pm n}\) along the string – the \(n\)-th Fourier component in the expansion of a combination of \(x^{\prime \mu}(\sigma)\) and \(p^\mu(\sigma)\) – and \(\alpha_n,\alpha_{-n}\) obey the algebra of annihilation and creation operators, respectively.
A general excited open string state is obtained by the action of these harmonics on the ground state (usually a tachyonic ground state) \(\ket 0\):\[
\dots (\alpha_{-3})^{N_3} (\alpha_{-2})^{N_2} (\alpha_{-1})^{N_1} \ket 0
\] where the exponents \(N_j\) are non-negative integers (only finitely many are nonzero). For each higher harmonic, the string may be excited by the corresponding vibration – an integer number of times because the string obeys the laws of quantum mechanics and the quantum harmonic oscillator has an equally spaced spectrum. Such an excited string behaves as a particle whose mass is proportional to\[
m^2 = m_0^2 (\dots + 3N_3 + 2N_2 + 1N_1 - 1)
\] The more excitations you include, the heavier particle you get. The higher harmonics increase the string theory's mass more quickly. The term \(-1\) is a contribution from the zero-point energies of all these oscillators. You may derive this negative shift as a term proportional to the renormalized sum of integers\[
1+2+3+\dots \to -\frac{1}{12}
\] I've replaced \(=\) by \(\to\) just because I want to reduce the number of angry clueless critics by 70% but be sure that \(=\) would be more accurate.
The characteristic scale \(m\) may be close to the GUT scale if not the Planck scale. But there also exist low-string-scale models (brane worlds) where \(m\) is comparable to a few \({\rm TeV}\)s, the energies marginally accessible by the LHC. I was surprised that Tetragraviton didn't have a clue about the possibility of a low string scale.
In the formula above, I suppressed the \(\mu\) index so I was only adding vibrations in one transverse dimension. A realistic 10D superstring requires 8 copies of such oscillators, all of them may excite the string by the same amount, and there may also be similar fermionic oscillators living on the string. Their contributions to the masses are analogous – except that the corresponding operators "mostly anticommute" and the occupation numbers are therefore \(0\) or \(1\).
For closed strings, we have two sets of oscillators – left-moving and right-moving oscillators \(\alpha\) and \(\tilde\alpha\). Both of them may be added to excite the string. The total \(m^2\) calculated from the left-movers must agree with the total \(m^2\) calculated from the right-movers. The requirement is known as the level-matching condition, \(L_0=\tilde L_0\), and it is basically equivalent to the statement that the choice of the \(\sigma=0\) "origin" of a closed string must be unphysical (the total momentum along/around the closed string must vanish).
Note that our formula calculated \(m^2\) and not \(m\) as the integer. This is due to a rather elementary kinematic technicality that boils down to relativity. In relativity, things simplify when the strings are highly boosted or described in the "light cone gauge". In that case, the component \(p^-\) of the energy-momentum vector – a light-cone gauge edition of "energy" – turns out to contain a term proportional to \(m^2\). (Explanations without the light-cone gauge are possible, too.)
You may have been afraid that in relativity, the energy formula would unavoidably contain lots of square roots from \(E=\sqrt{M^2+P^2}\) which would make all the oscillators unharmonic. But this trap may be avoided by a choice of coordinates on the world sheet. In particular, in the light-cone gauge (really a conformal gauge is enough), the internal energy of the string is linked to \(m^2\) of the corresponding particle and the formula for \(m^2\) reduces to simple harmonic oscillators without square roots. None of these things may be clear to anyone "without any calculations" but the students learn and verify the reasons before the 5th lecture of string theory. The result is that even though the string is a relativistic object (the vibration equations are Lorentz-covariant), the relevant Hamiltonians may be written by simple formulae involving harmonic oscillators and no square roots.
So in some units, the squared masses \(m^2\) of allowed vibrating strings are literally integers in certain units.
The squared masses of known particle species are not equally spaced in this way. It's mostly because
Extra dimensions may be flat (torus or its orbifolds) and supersymmetry may be expressed in terms of free fields (whose spectrum is exactly gotten by adding the energy of the higher harmonics).
Moreover, Tetragraviton's extra comments about extra dimensions and supersymmetry are absolutely demagogic given the fact that he claimed to show something inaccurate about Brian Greene's statements about string theory. Brian Greene has always discussed extra dimensions and supersymmetry in much more detail than Tetragraviton. For example, several full chapters are dedicated to these topics in The Elegant Universe.
I want to emphasize that there are actually semirealistic models of string theory – which basically produce the minimal supersymmetric standard model or something like that consistently coupled to quantum gravity – which still build the spectrum pretty much by the simple addition of the higher harmonics that I discussed above. In particular, I mean the orbifolds of tori and the heterotic models in the free fermionic formulation.
A novelty of such orbifolds is that some of the states come from twisted sectors. A twisted sector has some new boundary conditions. A round trip around the closed string doesn't return you to the same point in the space (or configuration space) but one related by a global symmetry (isometry of the compactification manifold or a generalization of an isometry). Consequently, the indices \(n\) of \(\alpha_{n}\) are no longer integers but they are shifted by a fractional shift such as \(1/2\) or \(1/4\) away from an integer. This isn't changing the story qualitatively. It's still true that the squared masses are integer multiples of a quantum. Also, the negative additive shift in the formula for \(m^2\) – the ground state energy – depends on which (twisted – or untwisted) sector you consider.
Let me discuss Tetragraviton's claims in more detail.
Experimentally, we have only observed a few dozens of particle species. But they come from the string tower of vibrating strings, too. In some approximation, they're usually coming from states with \(m^2=0\). But that does not mean that the counting of the higher harmonics and their contributions to \(m^2\) may be avoided.
Why?
It's because of the negative shift in the \(m^2\). The ground state of a (closed or open) string is normally a tachyon with \(m^2\lt 0\). This state is projected out by the so-called GSO projection. At the end, the spacetime supersymmetry is a sufficient (but not necessary!) condition to get rid of all the tachyons. But there are always numerous massless states – in the approximation of free strings. And these states are massless because the negative ground state contribution to \(m^2\) is cancelled by the positive contributions from the oscillators. This cancellation may take a different numerical form for different states – and especially for states in different twisted sectors.
But again, the counting of the basic frequency's and higher harmonics' energy is unavoidable even if you want to understand the origin of the massless states. If all the non-constant modes along the string could be completely ignored and omitted, the whole added value of string theory would be "redundant garbage" and we could just work with the equally consistent massless truncation of string theory.
However, we just can't. In particular, one of the massless i.e. \(m^2=0\) states of the vibrating string is the graviton, the quantum of the gravitational wave (or field), the messenger of the gravitational force. Even in the simplest \(D=26\) bosonic string theory, the spin-two graviton states are obtained from a closed string by the action of two oscillators:\[
\alpha^\mu_{-1} \tilde \alpha^\nu_{-1} \ket{0}
\] Similarly, in the RNS \(D=10\) definition of superstring theory, it is\[
\alpha^\mu_{-1/2} \tilde \alpha^\nu_{-1/2} \ket{0}_{NS,NS}
\] The ground state is a tachyon (which survives in bosonic string theory, a source of infrared inconsistencies equivalent to an instability, but is removed by the GSO projections in superstring theory). But its negative \(m^2\) is exactly cancelled by one left-moving excitation of the "basic frequency wave" on the string, and one right-moving one (note that the level-matching condition holds). There is no way to get the same results without the non-constant "sinusoidal waves" on the string.
The point that 4gravitons is missing is that massless states (in the free-string approximation) coming from the quantized strings are massless "by accident". Most states have positive masses, some states happen to have zero masses when the terms are added. But the latter aren't separated from the rest in any a priori way. The massive excitations are in no way added artificially to some massless starting point. There is no massless starting point in string theory. String theory unavoidably generates the massless and massive states simultaneously, with no consistent way to divide them. It is not quite trivial to derive the massless spectrum in a general string compactification. It's about as hard as to derive the states at any massive level.
OK, back to the graviton state that had two (minimal nonzero frequency) wave excitations around the closed string.
Once you allow the "basic frequency" of the wave on the string, you automatically allow all of them because splitting and joining strings are capable of producing truncated sines on a shorter interval which may only be Fourier-expanded on the shorter string if you allow all the higher harmonics as well. And a consistent theory of quantum gravity may only be obtained if you incorporate all of them. There's just no way to consistently truncate the higher harmonics because even the "simple" graviton depends on the non-constant modes along the string.
But I believe that this is not the only problem with his views about string theory. Another paragraph says:
But it's primarily the following paragraph that I believe to be seriously flawed:
But the generalization of this statement, "they [strings excited by the harmonics] are not how string theory explains the fundamental particles of nature" is surely the opposite of a deep truth. That is exactly how string theory explains the fundamental particles of Nature. Barton Zwiebach's quote above may be used as the best explanation in this blog post why this observation is both right and essential.
There may also be some confusion about "what counts as a fundamental particle of Nature". Tetragraviton seems to count the electron but not some heavy states near the string scale. But both of them are fundamental particles of Nature. Moreover, both of them have masses whose essential contribution comes from the energy of vibrations added to the string. There is no qualitative difference between the electron and the graviton; and heavier string states. We may have detected some particles and not others but all of them are equally real and equally fundamental.
But a point that he repeats all the time and that annoys me is one about the "particle you're used to". String theory isn't primarily a theory mean to discuss "just the particles you used to". String theory is a theory of everything – which includes all particles, including those that no one is used to because we haven't observed them (yet).
If someone isn't interested in the full list of particles in Nature, I find it obvious that he has no reason to be interested in string theory, either – because string theory is almost by definition a theory going well beyond the technical limitations of current experiments. If someone isn't interested what is hiding beneath the surface, an effective field theory is an easier attitude for those whose interest is this narrow-minded. The effective field theories are really defined to be the answer to the questions that never try to go beyond a certain regime defined by practical limitations. But in that case, if someone is interested in these low-energy things only, I don't see why he would be reading blog posts or books about string theory at all.
It just makes no sense whatsoever. The person isn't interested in these questions so he probably doesn't study them and hasn't studied them. He almost certainly knows nothing about the things he could have learned (about string theory) but he hasn't and he should better shut his mouth.
Quite generally, a popularizer of science always runs the risk of being separated from the big shots who do the best research, and so on. People realize this (true) general fact and that's also why popularizers are sometimes attacked with similar words. However, in the case of string theory, almost all these attacks are just plain rubbish.
In particular, Brian Greene has been extremely careful what he was saying about string theory. His explanations of these topics correspond pretty much to the most accurate sketch that is accessible to a large enough subset of the lay public. And people who are criticizing some basic claims such as the deep insight that "in string theory, particles are vibrations" are simply full of šit. The identification of the particle species (all of them) and the vibration states of a string is a profound truth (of weakly coupled string theory).
Tetragraviton is a postdoc at the Perimeter Institute who has written several papers on multiloop amplitudes in gauge theory. Even though none of these papers depends on string theory in any tangible way, I've thought that he's a guy close enough to string theory who could potentially work on it which is why I was surprised by his blog post a week ago,
Particles Aren’t Vibrations (at Least, Not the Ones You Think)which indicates that I was wrong. The first sentence tells you what kind of popularizers are supposed to be a target:
You’ve probably heard this story before, likely from Brian Greene.I was imagining that there was something subtle. People may dislike the overabundant comments about "music and string theory" etc. But I didn't find anything too subtle in the blog post. While there's always some room for interpretations what a somewhat vague sentence addressed to the laymen could have meant, I think it's right to conclude that Tetragraviton is just flatly wrong.
Needless to say, the claim that (in weakly coupled string theory) different particle species are vibration modes of a string isn't just some fairy-tale used by Brian Greene. It's a translation of an actual defining fact of string theory into plain English. Brian Greene has in no way a monopoly over such a thing. Pretty much everyone else who has talked about string theory agrees that this is the right summary of string theory's ingenious description of the diversity of particle species.
Clearly, you may add people like Michio Kaku:
In string theory, all particles are vibrations on a tiny rubber band; physics is the harmonies on the string; chemistry is the melodies we play on vibrating strings; the universe is a symphony of strings, and the 'Mind of God' is cosmic music resonating in 11-dimensional hyperspace.Kaku and even Greene may sometimes be presented as "just some popularizers". But they have done highly nontrivial contributions to the field, too. And almost all other string theorists who talk about string theory use very similar formulations. I could give you dozens of examples. But because of his widely respected technical credentials, let me pick Edward Witten:
String theory is an attempt at a deeper description of nature by thinking of an elementary particle not as a little point but as a little loop of vibrating string. One of the basic things about a string is that it can vibrate in many different shapes or forms, which gives music its beauty. If we listen to a tuning fork, it sounds harsh to the human ear. And that's because you hear a pure tone rather than the higher overtones that you get from a piano or violin that give music its richness and beauty.The fact that particle species are types of vibrations isn't just a truth. It's pretty much "the defining truth", the very reason why string theory is unifying forces and matter. If you allow me to quote Barton Zwiebach's undergraduate textbook, A First Course in String Theory:
So in the case of one of these strings it can oscillate in many different forms—analogously to the overtones of a piano string. And those different forms of vibration are interpreted as different elementary particles: quarks, electrons, photons. All are different forms of vibration of the same basic string. Unity of the different forces and particles is achieved because they all come from different kinds of vibrations of the same basic string. In the case of string theory, with our present understanding, there would be nothing more basic than the string.
Why is string theory a truly unified theory? The reason is simple and goes to the heart of the theory. In string theory, each particle is identified as a particular vibrational mode of an elementary microscopic string. A musical analogy is very apt. Just as a violin string can vibrate in different modes and each mode corresponds to a different sound, the modes of vibration of a fundamental string can be recognized as the different particles we know. One of the vibrational states of strings is the graviton, the quantum of the gravitational field. Since there is just one type of string, and all particles arise from string vibrations, all particles are naturally incorporated into a single theory. When we think in string theory of a decay process...Everyone who understands string theory agrees with the essence of the statement that string theory explains particles as vibrations.
It's always amazing to see how many people like to pick an important truth, completely negate it, and claim that the result is a very important truth. It looks like they want to prove Niels Bohr's famous quote
The opposite of a correct statement is a false statement. But the opposite of a profound truth may well be another profound truth.Well, he only says that the opposite of a profound truth may be another profound truth. It usually isn't.
OK, so how did Tetragraviton argue that particles aren't vibrations?

We were shown the higher harmonics on a string with a claim that this is not how string theory produces the list of particle species. Except that it is a totally valid sketch of how string theory does it.
In a flat spacetime background, a single string really has possible higher harmonics \(\alpha^\mu_{\pm n}\) along the string – the \(n\)-th Fourier component in the expansion of a combination of \(x^{\prime \mu}(\sigma)\) and \(p^\mu(\sigma)\) – and \(\alpha_n,\alpha_{-n}\) obey the algebra of annihilation and creation operators, respectively.
A general excited open string state is obtained by the action of these harmonics on the ground state (usually a tachyonic ground state) \(\ket 0\):\[
\dots (\alpha_{-3})^{N_3} (\alpha_{-2})^{N_2} (\alpha_{-1})^{N_1} \ket 0
\] where the exponents \(N_j\) are non-negative integers (only finitely many are nonzero). For each higher harmonic, the string may be excited by the corresponding vibration – an integer number of times because the string obeys the laws of quantum mechanics and the quantum harmonic oscillator has an equally spaced spectrum. Such an excited string behaves as a particle whose mass is proportional to\[
m^2 = m_0^2 (\dots + 3N_3 + 2N_2 + 1N_1 - 1)
\] The more excitations you include, the heavier particle you get. The higher harmonics increase the string theory's mass more quickly. The term \(-1\) is a contribution from the zero-point energies of all these oscillators. You may derive this negative shift as a term proportional to the renormalized sum of integers\[
1+2+3+\dots \to -\frac{1}{12}
\] I've replaced \(=\) by \(\to\) just because I want to reduce the number of angry clueless critics by 70% but be sure that \(=\) would be more accurate.
The characteristic scale \(m\) may be close to the GUT scale if not the Planck scale. But there also exist low-string-scale models (brane worlds) where \(m\) is comparable to a few \({\rm TeV}\)s, the energies marginally accessible by the LHC. I was surprised that Tetragraviton didn't have a clue about the possibility of a low string scale.
In the formula above, I suppressed the \(\mu\) index so I was only adding vibrations in one transverse dimension. A realistic 10D superstring requires 8 copies of such oscillators, all of them may excite the string by the same amount, and there may also be similar fermionic oscillators living on the string. Their contributions to the masses are analogous – except that the corresponding operators "mostly anticommute" and the occupation numbers are therefore \(0\) or \(1\).
For closed strings, we have two sets of oscillators – left-moving and right-moving oscillators \(\alpha\) and \(\tilde\alpha\). Both of them may be added to excite the string. The total \(m^2\) calculated from the left-movers must agree with the total \(m^2\) calculated from the right-movers. The requirement is known as the level-matching condition, \(L_0=\tilde L_0\), and it is basically equivalent to the statement that the choice of the \(\sigma=0\) "origin" of a closed string must be unphysical (the total momentum along/around the closed string must vanish).
Note that our formula calculated \(m^2\) and not \(m\) as the integer. This is due to a rather elementary kinematic technicality that boils down to relativity. In relativity, things simplify when the strings are highly boosted or described in the "light cone gauge". In that case, the component \(p^-\) of the energy-momentum vector – a light-cone gauge edition of "energy" – turns out to contain a term proportional to \(m^2\). (Explanations without the light-cone gauge are possible, too.)
You may have been afraid that in relativity, the energy formula would unavoidably contain lots of square roots from \(E=\sqrt{M^2+P^2}\) which would make all the oscillators unharmonic. But this trap may be avoided by a choice of coordinates on the world sheet. In particular, in the light-cone gauge (really a conformal gauge is enough), the internal energy of the string is linked to \(m^2\) of the corresponding particle and the formula for \(m^2\) reduces to simple harmonic oscillators without square roots. None of these things may be clear to anyone "without any calculations" but the students learn and verify the reasons before the 5th lecture of string theory. The result is that even though the string is a relativistic object (the vibration equations are Lorentz-covariant), the relevant Hamiltonians may be written by simple formulae involving harmonic oscillators and no square roots.
So in some units, the squared masses \(m^2\) of allowed vibrating strings are literally integers in certain units.
The squared masses of known particle species are not equally spaced in this way. It's mostly because
- the strings generally vibrate in a curved spacetime background
- the particles – vibrations of strings – interact with each other (because strings split and join) and this has a similar effect on the masses as field theory phenomena such as the Higgs mechanism; in fact, the Higgs mechanism and all similar things work in string theory "just like" in field theory
Extra dimensions may be flat (torus or its orbifolds) and supersymmetry may be expressed in terms of free fields (whose spectrum is exactly gotten by adding the energy of the higher harmonics).
Moreover, Tetragraviton's extra comments about extra dimensions and supersymmetry are absolutely demagogic given the fact that he claimed to show something inaccurate about Brian Greene's statements about string theory. Brian Greene has always discussed extra dimensions and supersymmetry in much more detail than Tetragraviton. For example, several full chapters are dedicated to these topics in The Elegant Universe.
I want to emphasize that there are actually semirealistic models of string theory – which basically produce the minimal supersymmetric standard model or something like that consistently coupled to quantum gravity – which still build the spectrum pretty much by the simple addition of the higher harmonics that I discussed above. In particular, I mean the orbifolds of tori and the heterotic models in the free fermionic formulation.
A novelty of such orbifolds is that some of the states come from twisted sectors. A twisted sector has some new boundary conditions. A round trip around the closed string doesn't return you to the same point in the space (or configuration space) but one related by a global symmetry (isometry of the compactification manifold or a generalization of an isometry). Consequently, the indices \(n\) of \(\alpha_{n}\) are no longer integers but they are shifted by a fractional shift such as \(1/2\) or \(1/4\) away from an integer. This isn't changing the story qualitatively. It's still true that the squared masses are integer multiples of a quantum. Also, the negative additive shift in the formula for \(m^2\) – the ground state energy – depends on which (twisted – or untwisted) sector you consider.
Let me discuss Tetragraviton's claims in more detail.
It’s a nice story. It’s even partly true. But it [the claim that increasingly heavy particle species are obtained from the addition of higher harmonics etc.] gives a completely wrong idea of where the particles we’re used to come from.Sorry but it gives a completely correct qualitative idea where all particle species in string theory come from. All of them come from string vibrations and it's always the case that (as long as one ignores subleading corrections to the masses from field theory effects etc.) the more vibrations are added to a string, the heavier particle species we obtain.
Experimentally, we have only observed a few dozens of particle species. But they come from the string tower of vibrating strings, too. In some approximation, they're usually coming from states with \(m^2=0\). But that does not mean that the counting of the higher harmonics and their contributions to \(m^2\) may be avoided.
Why?
It's because of the negative shift in the \(m^2\). The ground state of a (closed or open) string is normally a tachyon with \(m^2\lt 0\). This state is projected out by the so-called GSO projection. At the end, the spacetime supersymmetry is a sufficient (but not necessary!) condition to get rid of all the tachyons. But there are always numerous massless states – in the approximation of free strings. And these states are massless because the negative ground state contribution to \(m^2\) is cancelled by the positive contributions from the oscillators. This cancellation may take a different numerical form for different states – and especially for states in different twisted sectors.
But again, the counting of the basic frequency's and higher harmonics' energy is unavoidable even if you want to understand the origin of the massless states. If all the non-constant modes along the string could be completely ignored and omitted, the whole added value of string theory would be "redundant garbage" and we could just work with the equally consistent massless truncation of string theory.
However, we just can't. In particular, one of the massless i.e. \(m^2=0\) states of the vibrating string is the graviton, the quantum of the gravitational wave (or field), the messenger of the gravitational force. Even in the simplest \(D=26\) bosonic string theory, the spin-two graviton states are obtained from a closed string by the action of two oscillators:\[
\alpha^\mu_{-1} \tilde \alpha^\nu_{-1} \ket{0}
\] Similarly, in the RNS \(D=10\) definition of superstring theory, it is\[
\alpha^\mu_{-1/2} \tilde \alpha^\nu_{-1/2} \ket{0}_{NS,NS}
\] The ground state is a tachyon (which survives in bosonic string theory, a source of infrared inconsistencies equivalent to an instability, but is removed by the GSO projections in superstring theory). But its negative \(m^2\) is exactly cancelled by one left-moving excitation of the "basic frequency wave" on the string, and one right-moving one (note that the level-matching condition holds). There is no way to get the same results without the non-constant "sinusoidal waves" on the string.
The point that 4gravitons is missing is that massless states (in the free-string approximation) coming from the quantized strings are massless "by accident". Most states have positive masses, some states happen to have zero masses when the terms are added. But the latter aren't separated from the rest in any a priori way. The massive excitations are in no way added artificially to some massless starting point. There is no massless starting point in string theory. String theory unavoidably generates the massless and massive states simultaneously, with no consistent way to divide them. It is not quite trivial to derive the massless spectrum in a general string compactification. It's about as hard as to derive the states at any massive level.
OK, back to the graviton state that had two (minimal nonzero frequency) wave excitations around the closed string.
Once you allow the "basic frequency" of the wave on the string, you automatically allow all of them because splitting and joining strings are capable of producing truncated sines on a shorter interval which may only be Fourier-expanded on the shorter string if you allow all the higher harmonics as well. And a consistent theory of quantum gravity may only be obtained if you incorporate all of them. There's just no way to consistently truncate the higher harmonics because even the "simple" graviton depends on the non-constant modes along the string.
Again, even for massless states, the careful counting of the energy from nontrivial sinusoidal excitations of the string is essential to get the correct mass.Disappointingly, the only interpretation of Tetragraviton's words that "the vibrating string picture with harmonics is a completely wrong explanation of the well-known particle species" is that he just doesn't have a clue how string theory explains the massless and light states.
But I believe that this is not the only problem with his views about string theory. Another paragraph says:
String theory’s strings are under a lot of tension, so it takes a lot of energy to make them vibrate. From our perspective, that energy looks like mass, so the more complicated harmonics on a string correspond to extremely massive particles, close to the Planck mass!I've discussed that, it's not necessarily the case. I do believe that the string scale is close to the Planck mass but there do exist low-string models where it's as low as a few \({\rm TeV}\)s. This is just a technical difference. The heavier excited string vibrations are equally real in both scenarios.
But it's primarily the following paragraph that I believe to be seriously flawed:
Those aren’t the particles you’re used to. They’re not electrons, they’re not dark matter. They’re particles we haven’t observed, and may never observe. They’re not how string theory explains the fundamental particles of nature.Electrons and particles of dark matter (if the latter is composed of particles) are excited strings as well, and even for those, the addition of energies from vibrations on the string is needed to get the correct mass (despite its being zero in the free-string approximation). There just doesn't exist any sense in which the statement that "the states in the infinite tower of arbitrarily excited strings don't describe the electron or dark matter" could be correct. It's just wrong, wrong, wrong.
But the generalization of this statement, "they [strings excited by the harmonics] are not how string theory explains the fundamental particles of nature" is surely the opposite of a deep truth. That is exactly how string theory explains the fundamental particles of Nature. Barton Zwiebach's quote above may be used as the best explanation in this blog post why this observation is both right and essential.
There may also be some confusion about "what counts as a fundamental particle of Nature". Tetragraviton seems to count the electron but not some heavy states near the string scale. But both of them are fundamental particles of Nature. Moreover, both of them have masses whose essential contribution comes from the energy of vibrations added to the string. There is no qualitative difference between the electron and the graviton; and heavier string states. We may have detected some particles and not others but all of them are equally real and equally fundamental.
So how does string theory go from one fundamental type of string to all of the particles in the universe, if not through these vibrations? As it turns out, there are several different ways it can happen. I’ll describe a few.Again, it's simply not true that supersymmetry replaces or invalidates the fact that the main contribution to the mass of particles in string theory comes from the vibrations of a string. Supersymmetry is a special feature of a subset of the string vacua (and similarly quantum field theories). But the elements of this subset are constructed in the same way as elements outside this subset. In string theory, they are constructed by counting the energy that vibrations on a quantum relativistic string may carry. Supersymmetry almost always requires some fermionic degrees of freedom but they may be viewed as extra coordinates of the (super)space and they add vibrations and energy through (fermionic) harmonic oscillators just like their bosonic friends (well, they're not just Platonic friends, they're superpartners). They also have higher harmonics with \(n=2,3\) etc., only the occupation numbers are \(N_a=0,1\).
The first and most important trick here is supersymmetry. ...
Supersymmetry relates different types of particles to each other. In string theory, it means that along with vibrations that go higher and higher, there are also low-energy vibrations that behave like different sorts of particles.Supersymmetry makes it more likely that there will be massless or light particles but it is not a necessary condition. There exist non-supersymmetric (yet tachyon-free) string vacua with the analogous massless portion of the spectrum (massless is meant at the level of the free string, the string scale). Despite the absence of supersymmetry, the number of massless bosonic and fermionic particle species – massless states of a vibrating string – is basically the same as in the similar supersymmetric models (I am talking about the tachyon-free non-SUSY heterotic strings). The states are just different, not less numerous or "worse".
Even with supersymmetry, string theory doesn’t give rise to all of the right sorts of particles. You need something else, like compactifications or branes.Yup, except that Brian Greene and many others have explained all these things with extra dimensions etc. far more accurately and pedagogically than Tetragraviton. Incidentally, a compactification is always needed to obtain at least a semi-realistic string vacuum.
In string theory, the particles we’re used to aren’t just higher harmonics, or vibrations with more and more energy. They come from supersymmetry, from compactifications and from branes.Again, there is absolutely no "contradiction" between vibrations on one side and compactifications, SUSY, or branes on the other side. They're independent concepts. Strings vibrate even when they're placed in a compactified spacetime manifold, even when they're supersymmetric, even when there are D-branes around, and even if the strings are open strings attached to these D-branes. It's similar to the bass strings: they also vibrate even when they are surrounded by an instrument whose shape resembles Meghan Trainor, no treble. The shape of the instrument, like the shape of the compactification manifold, influences the sound (and spectrum) of the vibrations but it in no way invalidates or removes the vibrations.
But a point that he repeats all the time and that annoys me is one about the "particle you're used to". String theory isn't primarily a theory mean to discuss "just the particles you used to". String theory is a theory of everything – which includes all particles, including those that no one is used to because we haven't observed them (yet).
If someone isn't interested in the full list of particles in Nature, I find it obvious that he has no reason to be interested in string theory, either – because string theory is almost by definition a theory going well beyond the technical limitations of current experiments. If someone isn't interested what is hiding beneath the surface, an effective field theory is an easier attitude for those whose interest is this narrow-minded. The effective field theories are really defined to be the answer to the questions that never try to go beyond a certain regime defined by practical limitations. But in that case, if someone is interested in these low-energy things only, I don't see why he would be reading blog posts or books about string theory at all.
It just makes no sense whatsoever. The person isn't interested in these questions so he probably doesn't study them and hasn't studied them. He almost certainly knows nothing about the things he could have learned (about string theory) but he hasn't and he should better shut his mouth.
The higher harmonics are still important: there are theorems that you can’t fix quantum gravity with a finite number of extra particles, so the infinite tower of vibrations allows string theory to exploit a key loophole.Right. All the excited string modes are totally needed for the consistency of the quantum gravity, as I said. Also, as I discussed in a comment on the 4gravitons blog, when you gradually increase the value of the string coupling constant, the excited string states are gradually turning to black hole microstates. The exponential increase of the number of excited string states is a precursor or an approximation to the quasi-exponential increase of the number of black hole microstates we need in a consistent quantum theory of gravity.
They just don’t happen to be how string theory gets the particles of the Standard Model.If the world is described by a weakly coupled string theory, string theory does derive all the particles of the Standard Model exactly by the same algorithm that 4gravitons irrationally denounces.
The idea that every particle is just a higher vibration is a common misconception, and I hope I’ve given you a better idea of how string theory actually works.Is it not a misconception and Tetragraviton has only brought confusion and falsehoods to this topic.
Quite generally, a popularizer of science always runs the risk of being separated from the big shots who do the best research, and so on. People realize this (true) general fact and that's also why popularizers are sometimes attacked with similar words. However, in the case of string theory, almost all these attacks are just plain rubbish.
In particular, Brian Greene has been extremely careful what he was saying about string theory. His explanations of these topics correspond pretty much to the most accurate sketch that is accessible to a large enough subset of the lay public. And people who are criticizing some basic claims such as the deep insight that "in string theory, particles are vibrations" are simply full of šit. The identification of the particle species (all of them) and the vibration states of a string is a profound truth (of weakly coupled string theory).
Particles are vibrations
![Particles are vibrations]() Reviewed by MCH
on
May 18, 2016
Rating:
Reviewed by MCH
on
May 18, 2016
Rating:



No comments: