After some time, motivated by a new package of misunderstanding and mystification that has spread across the blogosphere, I decided to write a text about the black hole information puzzle.
Previous articles about the same issue:
The black holes have been known to exist, according to general relativity, for more than 50 years, long before they were located by astronomers. Black holes seem to be able to "eat" objects and "destroy" the information in these objects. What is left is a typically spherical black object with "no hair" that doesn't seem to remember anything about the initial state.
Before 1974, you could have hoped that the information about the initial matter is preserved inside the black hole: it is inaccessible but it is there. However, in 1974, Stephen Hawking discovered that black holes evaporate. The radiation coming from the black hole is exactly thermal and doesn't seem to carry any information either - a point that will be clarified later. At the end, there is no black hole interior left and there is no room where the information could be hiding. Once the black hole evaporates completely, the information simply seems to be lost entirely.
Why is it bad?
In conventional classical physics as well as quantum physics, there is a one-to-one correspondence between the (initial) state of the system at t=t_0 and its (final) state at t=t_1.
In quantum physics, the states are vectors in a linear Hilbert space and the one-to-one correspondence, as dictated by the Schrödinger equation, also requires the probabilities of the initial state and the corresponding final state to coincide. This condition is mathematically expressed as the unitarity of the evolution operator. The evolution must be a complexified version of an orthogonal transformation - one that preserves the length of vectors.
So according to quantum mechanics and its holy principle of unitarity, the information about the initial object (star...) must be preserved somewhere after the black hole evaporates. However, that's impossible according to general relativity because no particle - and even no information - is allowed to escape from the interior of the black hole. Such escaping information would be as bad as superluminal signals that you may have discussed in the context of special relativity. We say that such an event would violate causality, the principle that an event can only be influenced by its past (and in relativity, only by events in its past light cone).
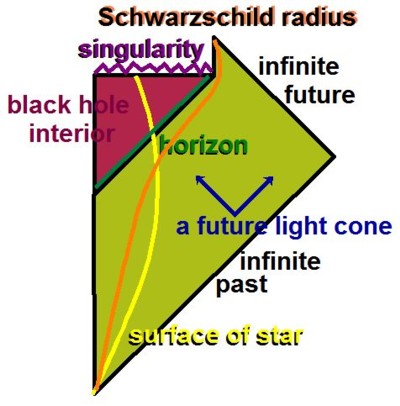
A Penrose (causal) diagram of an evaporating black hole. You can't trust the distances but you can trust the angles in the "r-t" plane: world lines of light are always 45° diagonals. Each point of the diagram is a two-sphere in spacetime. A star that is too heavy and shrinks under the appropriate Schwarzschild radius (orange curve) inevitably collapses. Unlucky observers on the yellow surface don't even notice but at some moment they cross the green diagonal horizon, a (null) hypersurface separating the interior (the purple triangle) from the (light green) exterior. The information can't get from the interior to the exterior, especially not to the "infinite future", another diagonal line, because you would need a nearly horizontal (superluminal) trajectory for that. All material world lines that ever occur inside the black hole are guaranteed to hit the wiggly singularity sometime in the future and they are destroyed by huge curvature over there. What happens near the singularity depends on details of quantum gravity but it doesn't influence the "rough" structure of the causal diagram - one that can be derived purely from (experimentally verified) low-energy Einstein's equations, assuming that they hold in all regions with an allowed exception near the singularity.
So we seem to have a direct contradiction between [QM and unitarity] and [GR and causality]. Both of these principles, unitarity and causality, cannot be exactly correct because a contradiction arises from their explosive mixture.
What does it mean to solve the problem?
A solution to the apparent paradox is to have a self-consistent theory and a self-consistent set of interpretations and partial, at least qualitative answers that
The apparent paradox only arises if we take these established principles of physics into account. If you deny these principles, it is not surprising that you can also avoid the paradox. But such a denial solves nothing for the people who are aware of the principles that have led to the paradox in the first place. ;-)
It is of course possible that some of the fundamental assumptions of current physics - maybe even the very existence of black holes - is incorrect. But if it is so, a suggestion that assumes such a radical departure from the established insights of physics must also replace all the theoretical and experimental evidence supporting general relativity by an entirely new framework. If you just close your eyes, deny some results, and say that you don't have to explain why general relativity has been so accurate in other contexts, you are not solving any puzzle. OK?
The comments above imply that a solution must inevitably modify at least one of the following assumptions:
What's the correct solution?
As most quantum mechanicians have known from the very beginning, it is the unitarity, a principle of quantum mechanics, that wins in the battle and remains universally valid.
On the other hand, causality becomes an approximate principle that is only valid in the limit of infinitely large causal diamonds. In the presence of black holes, the internal causal structure is modified by quantum phenomena and the information can "tunnel" out of the black hole.
When you summarize all these effects, the radiation emitted by black holes is analogous to the radiation emitted by stars or other "burning" objects: it looks pretty thermal but the information about the "burned" matter is stored in the radiation in principle: it is encoded in subtle correlations between the photons, their precise directions, and their frequencies.
Why is it the correct solution?
It shouldn't be so surprising that unitarity survives completely while causality doesn't. After all, the basic postulates of quantum mechanics, including unitarity, the probabilistic interpretation of the amplitudes, and the linearity of the operators representing observables, seem to be universally necessary to describe physics of any system that agrees with the basic insights of the quantum revolution.
On the other hand, geometry has been downgraded into an effective, approximate, emergent aspect of reality. The metric tensor is just one among many fields in our effective field theories including gravity. In string theory, there are, in some sense, infinitely many such fields besides the metric tensor - the whole "stringy tower". The metric tensor doesn't have to exist as a good degree of freedom at the Planck scale or in other extreme conditions. We know many other fields that are only good enough at low energies - e.g. the pion field. Also, the Einstein-Hilbert action is just an approximation that is corrected by many corrections whose qualitative character is known. That's what we qualitatively know from all the lessons of the renormalization group, effective field theories, and so on.
The light cones needed to determine causal relations between events are calculated from the metric tensor. Because the latter is an approximate concept, it is not surprising that causality is approximate, too. In the context of compact, temporary objects such as black holes, you shouldn't be shocked that the causal separation between different regions of spacetime is an approximate notion, too.
Matter and information can tunnel in between different portions of the spacetime. The retrieval of the information is just another example of quantum tunneling. The Hawking radiation itself may be viewed as an example of quantum tunneling - a process that can break the classical limitations of space e.g. penetrate the wall. Such a process may also break causality, for a limited amount of time. That's why it is really possible, in a quantum theory of gravity, to extract the information from the black hole.
Equally importantly, we have understood - pretty accurately - the character of the theories that realize this solution to the black hole information puzzle. The situation is most obvious in Matrix theory and the AdS/CFT correspondence. In these two explicit methods to define physics of string theory, we can show that the rules of general relativity including Einstein's equations and the existence of black holes are satisfied. At the same moment, we can rigorously prove that the information is preserved because these systems may be described as an old-fashioned, non-gravitational quantum system governed by a Hermitean Hamiltonian.
The huge progress in the stringy understanding of black hole dynamics is probably the main reason why we are so certain that we (=competent physicists) know the correct answer to the black hole information puzzle. The information is preserved and the causality doesn't hold exactly in compact regions of spacetime.
It is somewhat harder to create a "fully satisfactory psychological model" showing the form of the information as it leaves the black hole and "mapping the detailed degrees of freedom" to each other. However, we are not really guaranteed that such an intuitive model, with a comprehensible description "where the information goes" when it leaves the black hole, must exist. In the context of ordinary quantum tunneling, you can't really reconstruct the trajectory of the particle through the inpenetrable wall, either.
I want to say that while we know almost certainly what is the correct answer to the black hole information puzzle, our knowledge is not really a "rigorous proof". After all, we're dealing with a natural science, not with mathematics, and no fully rigorous proofs about the real world can ever exist. It means that we're not really saying that it is 100.00000000% impossible for the Universe to work according one of the contrived, unnatural, miraculous, alternative scenarios discussed below.
But if you remember Steven Weinberg's interview about religion, he said that science as pioneered by Galileo and Newton hasn't made religion impossible. Instead, it has made irreligion possible. The situation with the black hole information puzzle is analogous. The recent breakthroughs, including the detailed description of quantum gravity by Matrix theory and AdS/CFT correspondence, haven't rigorously proved that it is impossible for the real world to be losing information, employ black hole remnants, or do other bizarre things. Why? Because if any uncertainty is an issue for you, Matrix theory and the AdS/CFT (and string theory) don't have to describe the real world, not even qualitatively.
But what these breakthroughs have shown is that the Universe can work in a way that satisfies unitarity and that also satisfies the rules and conclusions of general relativity in the low-energy limit. With subtle - and normally undetectable - mechanisms such as "quantum tunneling" described above, the rules of quantum gravity seem to be perfectly consistent with everything that we know. So this picture - most accurately encoded in string theory - is a solution to the black hole information puzzle.
Once again, this doesn't really prove that there can't exist some other, totally different solutions. But it does show that these hypothetical alternative solutions would have to make a lot of progress to become real competitors whose likelihood could be compared to the likelihood of the string-theory-based answer.
Why are the wrong solutions unsatisfactory?
Guess: information is lost indeed: the evolution is non-unitary:
Sabine Hossenfelder wrote seven solutions and incorrectly claimed that all of them have advantages and disadvantages. From reading her general clichés, you have no method to figure out which solution(s) is (are) actually the correct one(s) and why. Her reasons to dislike the correct solution(s) are irrational, as argued below.
In fact, it is fair to say that two "proposed solutions" she listed are correct, namely that the evolution is unitary as known from the AdS/CFT correspondence; and the solution that the black holes do carry some (quantum) "hair", after all. They're both aspects of the same correct solution.
Hossenfelder claimed that every proposed solution has a "disadvantage" so she identifies a "problem" with the correct solutions, too. What's wrong with the solution that the evolution is known to be unitary from the AdS/CFT correspondence? She writes
She proposes to assume that the information must be getting lost and this conclusion may even be used to prove that the AdS/CFT correspondence is incorrect. Well, a subtle problem with the approach is that we can see that the AdS/CFT correspondence is valid and every existing argument that the AdS/CFT is invalid or incomplete has a known flaw. On the other hand, every "proof" that the information is lost is known to have a loophole. That's a damn asymmetric situation.
The very existence of the AdS/CFT dual theories is a proof that any "universal" proof that the information must be lost in quantum gravity is incorrect - simply because it is enough to have one counterexample and we have it. ;-) So what she's doing is just complaining that 2+2=4 shouldn't be correct because if she could prove that 1+1=3, it would also mean that 2+2=5. Indeed, one could probably construct such implications but the problem is that the main assumption, namely 1+1=3 (or information loss), cannot be established at a remotely comparable confidence level as the AdS/CFT correspondence. Not only it cannot be established: Hossenfelder's verbal exercise hasn't produced a glimpse of evidence, not even circumstantial or speculative evidence. You shouldn't blame a correct answer for merely being incompatible with the alternative, incorrect answers: that's what correct answers usually "do". ;-)
As far as her personal objection goes, it is ludicrous, too. While the AdS/CFT proof directly applies to anti de Sitter black holes only, the general conclusion that the information is preserved is surely much more general. For example, flat space may be obtained as a limit of a weakly curved AdS space. Moreover, black holes in certain flat spaces can be described in equally unitary Matrix theory, too.
In de Sitter space, the conservation of information is more subtle because even empty de Sitter space seems to have an "unpredictable" thermal radiation coming from the cosmic horizon. There is no "asymptotic region" of a de Sitter space and the information debates become more subtle. But they don't become more subtle because of black holes in the interior of the de Sitter space.
Black holes are localized objects and the behavior of these systems is surely universal: the local processes in string theory exhibit some properties that don't depend on remote regions - string theory is known to satisfy clustering, at least in some mild forms.
The only way how the asymptotic conditions of spacetime influence the conservation of energy is that
The AdS/CFT correspondence and Matrix theory are exactly unitary theories consistent with general relativity, the existence of black holes, and their evaporation in the low-energy limit and any proof that such a combination can't exist is wrong. Irreligion has become possible. Religion hasn't quite been proved impossible but it is no longer needed for the most rationally acceptable solution.
If you accept that the AdS black holes preserve the information but you claim that others don't, it's like accepting that Neil Armstrong used a spacecraft to get to the Moon but others will still have to walk. Jesus Christ: if we have already overcome the technical problems and created a spacecraft, why wouldn't we assume that other astronauts can use it, too? Again, I can't rigorously prove that you can't walk to the Moon, using this method. But I can see that the walking becomes a very contrived assumption once I know a gadget - the spacecraft - that can help you (and anyone) to get there.
Event horizons vs singularity: what defines a black hole
But later in her text, Sabine Hossenfelder exhibits her complete incompetence much more explicitly than by hiding the correct answers in the melting pot of five other answers that are known to be extremely unlikely. She also offers her own "idea" that is downright ludicrous. She claims that the essence of the paradox - and the key part of black holes in general - is not in the event horizon but in the singularity.
That's a misunderstanding shared by some undergraduate students who are just beginning to study physics of general relativity and who don't really understand the concepts well. It's analogous to young students of medicine who can't yet recognize stomachs from hearts. In reality, it is not the singularity but the event horizon that defines a black hole and it is the event horizon, not the singularity, that is relevant for the black hole information puzzle.
This statement, completely mysterious to Ms Hossenfelder, is completely elementary. Even the first sentence that defines a black hole at Wikipedia mentions the event horizon as a defining feature of black holes. The light cannot escape from the hole which is why it is (approximately) black. And it cannot escape because of the event horizon, not because of the singularity.
Why is the event horizon, and not the singularity, the crucial place that was responsible for the paradox? Simply because the general relativistic argument relevant for the paradox said that the information cannot escape from the black hole - that's how we know that it is lost. The region that separates the interior and the exterior is the event horizon. Its existence, combined with causality, makes it impossible for the information to get away.
When we talk about the possible information loss, we are comparing two slices through the whole spacetime: one slice occurs before the black hole is formed and another one occurs after the black hole evaporates. None of these slices contains any singularity which is why the singularity cannot be directly relevant for the paradox.
Complicated high-energy processes can take place and do take place near the singularity and the singularity is, most likely, not exactly "singular" when full physics of quantum gravity is taken into account. But that doesn't help us to solve the paradox. The information may be getting lost at the singularity, from the viewpoint of an observer who fell into the black hole and who is going to be destroyed, too.
But this unlucky observer is not the guy who defines the information loss paradox. It is not shocking that a person who is destroyed in a catastrophic gravitational field won't be able to reconstruct the information - because this guy has no optimistic future: in fact, he has no future at all. The information loss paradox arises when the past and future slices of the observer at infinity - outside the black hole - are compared with each other. Whether some unlucky observer inside the black hole thinks that the information was destroyed exactly when he died has no direct relevance for the question whether - and how - the information is lost from the asymptotic observer's viewpoint. The region around the singularity is localized and whatever happens there - and whichever "regularization" of the singularity the full theory chooses - has no impact on the conclusion that the information can't get away.
Summary
The black hole information puzzle is a textbook example of a theorist's puzzle par excellence. It is almost guaranteed that the exact information needed to reconstruct the initial state from the final state of the radiation will never be observed. So far, we haven't even observed the radiation itself. So this discussion has always been theoretical in character and it will probably remain forever theoretical.
But that doesn't mean that correct answers to such questions can't be found. Quite on the contrary, the black hole information puzzle is an example of a question where the correct answer is already known - the information is preserved and the causality is broken by tiny "tunneling" effects that make it possible for the information to escape.
While we know the correct answer, it is often difficult to pinpoint the exact problem of the previous ideas that have led to wrong answers. Why? Because these wrong answers usually assume the existence of degrees of freedom, terms in the action, and other objects that haven't been confirmed in the full theory of quantum gravity as we know it today - a description of a theory that is really equivalent to the phrase "string theory". In other words, these wrong solutions have made all kinds of assumptions that turned out to be incorrect.
In the case of fully defined superselections sectors of string theory - such as the models described by the AdS/CFT correspondence or Matrix theory - we know the states and the in-principle equations that transform the initial state to a final state. In many cases, it is difficult to "localize" the information contained in these states at particular points of space.
But that's just another, related lesson we have learned: although it can be shown that the low-energy approximation of string theory is local, the character of locality becomes subtle in extreme conditions, even in the presence of black holes. We can't really say "where the black hole hair is located" but we're not guaranteed that a good answer to this question exists in the first place. String theory implies the existence of many degrees of freedom but their precise association with a point in space is only guaranteed to exist if we avoid extreme deformations of space such as those that exist in the presence black holes. With black holes, degrees of freedom might be disconnected from particular points in space and if you (artificially) associate them with points in space, the resulting dynamics will be acausal and non-local.
Known and unknown things in physics
I am convinced that all competent quantum gravity physicists agree that the information is preserved in flat space or AdS space even though they may have a feeling that "we are still missing a part of the complete picture". Although you can formulate some arguments in favor of this proposition that don't depend on string theory, it is clear that string theory is by far the most important toolkit that makes us know that the information is preserved. It's also the main reason why Stephen Hawking has joined us to say that the information is preserved, after all.
One more thing to say: this story is a nice example of the huge explanatory power of string theory. While we may be uncertain which vacuum is the correct one - e.g. how many throats our Calabi-Yau space has if we live in one - there are many qualitative conclusions that string theory allows to make unequivocally. The conclusions about the preserved information are an example. They hold in all the 10^{500} vacua that are discussed under the trademark of the "landscape". These conclusions, and many others, are completely universal.
Black holes play a very special role in quantum gravity because they're the only "fundamental" localizable objects that are heavier than the fundamental scale. While the existence of strings and branes depends on the position in the landscape (or configuration space) and they are only useful concepts if some dimensionless moduli are much smaller than one, black holes are universal: they're also the only universal objects that are e.g. produced in all trans-Planckian collisions.
You may see that the people who don't like to study physics of quantum gravity carefully enough get completely lost even in very qualitative questions about physics. You ask them whether the information is lost and they return you 7 possible answers with 1 superficial paragraph per explanation why they dislike it. The explanations under the correct answers are manifestly wrong and many other explanations are confusing. You can simply see that the "quantum gravity theorists" outside the (broader) string theory have no idea what they're talking about.
In real science, it is crucial that one tries to divide the questions into the answered and unanswered ones as sharply and as rationally as possible. If someone can't answer any questions - e.g. not even the qualitative ones related to the information loss puzzle - her or his approach to physics has clearly made no progress. One can't ever be 100% certain but if you have no questions where your certainty clearly exceeds 90% or at least 50%, you have simply chosen a bad approach. You may have asked too ambitious questions and with no quasi-reliable answers, you have nothing to safely build upon.
The people in this bad situation often realize that they have found nothing and compensate this non-existing knowledge by "certainty" about all kinds of other, usually more detailed questions. Unfortunately, this "certainty" is based on pure guesswork and lacks any kind of rational justification. In most cases, the guesses are wrong. That's what guesses usually "do".
The actual cutting-edge status of physics is that many qualitative questions such as the question "is the information preserved?" have been answered while many detailed questions such as "how many throats our compact manifold has?" are uncertain. It is extremely counterproductive to force scientists to feel ashamed that there exist any open questions - such as the vacuum selection issues - because uncertainty and confusion is an inherent part of the scientific process that no scientist should ever be ashamed of. And it is equally counterproductive to force scientists to pretend the uncertainty about questions where they feel certain - such as the qualitative answers to the information loss puzzle.
Scientists should not only "try to know" but they should also "try to know what they know" and "try to know what they don't". It's bad to scream that known things should become unknown and everyone should try to build "diversity" about them, and it's equally bad to scream that unknown things shouldn't exist because a scientist who doesn't know something (or everything) is "not even wrong".
Various people who criticize theoretical physics are making these two complementary mistakes all the time which is too bad.
And that's the memo.
A fun historical paper: Stephen Hawking, Nature 248 (1974), Black hole explosions?
Previous articles about the same issue:
- Hawking and information loss
- Hawking and unitarity
- Black hole final state
- Two preprints on information loss
- Problems with black hole remnants
- Hayden+Preskill: fast information retrieval
The black holes have been known to exist, according to general relativity, for more than 50 years, long before they were located by astronomers. Black holes seem to be able to "eat" objects and "destroy" the information in these objects. What is left is a typically spherical black object with "no hair" that doesn't seem to remember anything about the initial state.
Before 1974, you could have hoped that the information about the initial matter is preserved inside the black hole: it is inaccessible but it is there. However, in 1974, Stephen Hawking discovered that black holes evaporate. The radiation coming from the black hole is exactly thermal and doesn't seem to carry any information either - a point that will be clarified later. At the end, there is no black hole interior left and there is no room where the information could be hiding. Once the black hole evaporates completely, the information simply seems to be lost entirely.
Why is it bad?
In conventional classical physics as well as quantum physics, there is a one-to-one correspondence between the (initial) state of the system at t=t_0 and its (final) state at t=t_1.
In quantum physics, the states are vectors in a linear Hilbert space and the one-to-one correspondence, as dictated by the Schrödinger equation, also requires the probabilities of the initial state and the corresponding final state to coincide. This condition is mathematically expressed as the unitarity of the evolution operator. The evolution must be a complexified version of an orthogonal transformation - one that preserves the length of vectors.
So according to quantum mechanics and its holy principle of unitarity, the information about the initial object (star...) must be preserved somewhere after the black hole evaporates. However, that's impossible according to general relativity because no particle - and even no information - is allowed to escape from the interior of the black hole. Such escaping information would be as bad as superluminal signals that you may have discussed in the context of special relativity. We say that such an event would violate causality, the principle that an event can only be influenced by its past (and in relativity, only by events in its past light cone).
A Penrose (causal) diagram of an evaporating black hole. You can't trust the distances but you can trust the angles in the "r-t" plane: world lines of light are always 45° diagonals. Each point of the diagram is a two-sphere in spacetime. A star that is too heavy and shrinks under the appropriate Schwarzschild radius (orange curve) inevitably collapses. Unlucky observers on the yellow surface don't even notice but at some moment they cross the green diagonal horizon, a (null) hypersurface separating the interior (the purple triangle) from the (light green) exterior. The information can't get from the interior to the exterior, especially not to the "infinite future", another diagonal line, because you would need a nearly horizontal (superluminal) trajectory for that. All material world lines that ever occur inside the black hole are guaranteed to hit the wiggly singularity sometime in the future and they are destroyed by huge curvature over there. What happens near the singularity depends on details of quantum gravity but it doesn't influence the "rough" structure of the causal diagram - one that can be derived purely from (experimentally verified) low-energy Einstein's equations, assuming that they hold in all regions with an allowed exception near the singularity.
So we seem to have a direct contradiction between [QM and unitarity] and [GR and causality]. Both of these principles, unitarity and causality, cannot be exactly correct because a contradiction arises from their explosive mixture.
What does it mean to solve the problem?
A solution to the apparent paradox is to have a self-consistent theory and a self-consistent set of interpretations and partial, at least qualitative answers that
- is (approximately?) consistent with unitarity in the context of quantum mechanical systems where unitarity is known to (approximately?) hold
- is consistent with the existence of black holes and (approximately?) with other conclusions of general relativity, including causality, where these conclusions are known to hold
- tells you, at least qualitatively, how these principles start to be modified (and when) in order to get rid of the contradiction
The apparent paradox only arises if we take these established principles of physics into account. If you deny these principles, it is not surprising that you can also avoid the paradox. But such a denial solves nothing for the people who are aware of the principles that have led to the paradox in the first place. ;-)
It is of course possible that some of the fundamental assumptions of current physics - maybe even the very existence of black holes - is incorrect. But if it is so, a suggestion that assumes such a radical departure from the established insights of physics must also replace all the theoretical and experimental evidence supporting general relativity by an entirely new framework. If you just close your eyes, deny some results, and say that you don't have to explain why general relativity has been so accurate in other contexts, you are not solving any puzzle. OK?
The comments above imply that a solution must inevitably modify at least one of the following assumptions:
- the evolution of quantum states is always and exactly unitary
- the interior of the black holes is always and completely causally disconnected from the exterior
What's the correct solution?
As most quantum mechanicians have known from the very beginning, it is the unitarity, a principle of quantum mechanics, that wins in the battle and remains universally valid.
On the other hand, causality becomes an approximate principle that is only valid in the limit of infinitely large causal diamonds. In the presence of black holes, the internal causal structure is modified by quantum phenomena and the information can "tunnel" out of the black hole.
When you summarize all these effects, the radiation emitted by black holes is analogous to the radiation emitted by stars or other "burning" objects: it looks pretty thermal but the information about the "burned" matter is stored in the radiation in principle: it is encoded in subtle correlations between the photons, their precise directions, and their frequencies.
Why is it the correct solution?
It shouldn't be so surprising that unitarity survives completely while causality doesn't. After all, the basic postulates of quantum mechanics, including unitarity, the probabilistic interpretation of the amplitudes, and the linearity of the operators representing observables, seem to be universally necessary to describe physics of any system that agrees with the basic insights of the quantum revolution.
On the other hand, geometry has been downgraded into an effective, approximate, emergent aspect of reality. The metric tensor is just one among many fields in our effective field theories including gravity. In string theory, there are, in some sense, infinitely many such fields besides the metric tensor - the whole "stringy tower". The metric tensor doesn't have to exist as a good degree of freedom at the Planck scale or in other extreme conditions. We know many other fields that are only good enough at low energies - e.g. the pion field. Also, the Einstein-Hilbert action is just an approximation that is corrected by many corrections whose qualitative character is known. That's what we qualitatively know from all the lessons of the renormalization group, effective field theories, and so on.
The light cones needed to determine causal relations between events are calculated from the metric tensor. Because the latter is an approximate concept, it is not surprising that causality is approximate, too. In the context of compact, temporary objects such as black holes, you shouldn't be shocked that the causal separation between different regions of spacetime is an approximate notion, too.
Matter and information can tunnel in between different portions of the spacetime. The retrieval of the information is just another example of quantum tunneling. The Hawking radiation itself may be viewed as an example of quantum tunneling - a process that can break the classical limitations of space e.g. penetrate the wall. Such a process may also break causality, for a limited amount of time. That's why it is really possible, in a quantum theory of gravity, to extract the information from the black hole.
Equally importantly, we have understood - pretty accurately - the character of the theories that realize this solution to the black hole information puzzle. The situation is most obvious in Matrix theory and the AdS/CFT correspondence. In these two explicit methods to define physics of string theory, we can show that the rules of general relativity including Einstein's equations and the existence of black holes are satisfied. At the same moment, we can rigorously prove that the information is preserved because these systems may be described as an old-fashioned, non-gravitational quantum system governed by a Hermitean Hamiltonian.
The huge progress in the stringy understanding of black hole dynamics is probably the main reason why we are so certain that we (=competent physicists) know the correct answer to the black hole information puzzle. The information is preserved and the causality doesn't hold exactly in compact regions of spacetime.
It is somewhat harder to create a "fully satisfactory psychological model" showing the form of the information as it leaves the black hole and "mapping the detailed degrees of freedom" to each other. However, we are not really guaranteed that such an intuitive model, with a comprehensible description "where the information goes" when it leaves the black hole, must exist. In the context of ordinary quantum tunneling, you can't really reconstruct the trajectory of the particle through the inpenetrable wall, either.
I want to say that while we know almost certainly what is the correct answer to the black hole information puzzle, our knowledge is not really a "rigorous proof". After all, we're dealing with a natural science, not with mathematics, and no fully rigorous proofs about the real world can ever exist. It means that we're not really saying that it is 100.00000000% impossible for the Universe to work according one of the contrived, unnatural, miraculous, alternative scenarios discussed below.
But if you remember Steven Weinberg's interview about religion, he said that science as pioneered by Galileo and Newton hasn't made religion impossible. Instead, it has made irreligion possible. The situation with the black hole information puzzle is analogous. The recent breakthroughs, including the detailed description of quantum gravity by Matrix theory and AdS/CFT correspondence, haven't rigorously proved that it is impossible for the real world to be losing information, employ black hole remnants, or do other bizarre things. Why? Because if any uncertainty is an issue for you, Matrix theory and the AdS/CFT (and string theory) don't have to describe the real world, not even qualitatively.
But what these breakthroughs have shown is that the Universe can work in a way that satisfies unitarity and that also satisfies the rules and conclusions of general relativity in the low-energy limit. With subtle - and normally undetectable - mechanisms such as "quantum tunneling" described above, the rules of quantum gravity seem to be perfectly consistent with everything that we know. So this picture - most accurately encoded in string theory - is a solution to the black hole information puzzle.
Once again, this doesn't really prove that there can't exist some other, totally different solutions. But it does show that these hypothetical alternative solutions would have to make a lot of progress to become real competitors whose likelihood could be compared to the likelihood of the string-theory-based answer.
Why are the wrong solutions unsatisfactory?
Guess: information is lost indeed: the evolution is non-unitary:
- Problems: no self-consistent "generalization" of quantum mechanics that would be non-unitary but that could include the well-established equations behind quantum systems is known. The postulates of quantum mechanics seem to be universally needed. Even if you only want to preserve the superposition principle but sacrifice unitarity, you are also forced to sacrifice clustering - the independence of separated regions of spacetime, a modest version of locality. Moreover, some well-known proposals to get a non-unitary picture require new boundary conditions in spacetime. These things break general covariance and consequently the energy conservation: the energy can get lost or produced at these new artificial boundaries. See e.g. Strominger 1994.
- Problems: this implies that infinitely many new particle species must exist. Arbitrarily high information can be squeezed into the Planckian volume - something that violates all the holographic bounds etc. Equivalently, such a new infinite number of localized species produces a lot of sick divergences in loop processes (e.g. drive the renormalized Newton's constant to zero), makes decay rates singular, and violates astrophysical bounds in the real Universe where too many unknown particles shouldn't exist. Analogous problems occur if you want to say that the whole information is emitted when the black hole is already tiny, near the end of the evaporation - because such a "new regime" of the evaporation is a version of the remnant that is physically small and carries the information. Finally, you haven't really saved unitarity because a pure state may evolve into a mixed state with the exactly thermal radiation that is a part of this answer.
- Problems: this is really a subset of the "information is indeed lost" approaches to the puzzle. If the information is preserved in a new segment of spacetime, the information carried by the two slices of the ordinary spacetime simply doesn't coincide: the information is lost according to the old rules. Talking about baby universes doesn't really help anything: in physics at least qualitatively related to the physics we know, all states can be described in terms of quasi-localized objects. Baby universes are degrees of freedom that are disconnected from any place in the usual spacetime: the physical interaction of the ordinary spacetime with these new un-localized degrees of freedom would probably violate locality and clustering. The baby universes are much like angels who can also be believed to carry the forgotten information. If we can't get the information from the angels, it is operationally lost. If we can talk to angels, it is not lost but the laws of physics become as non-local and superstitious as voodoo.
- Problems: as we explained in the introduction, these approaches can't be counted as solutions to anything unless you replace the whole "killed" branch of physics by entirely new and (at least equally) convincing explanations of the phenomena that were previously explained by the branch(es) of physics that you have "killed". For example, George Chapline would have to write completely new equations instead of Einstein's equations - equations that agree with the experiments verifying GR but that don't imply the existence of black holes. Most people who propose these truly revolutionary solutions are unfamiliar with the actual established physics and they simply ignore it. That's why they don't see any puzzle to start with and that's why their comments can't be viewed as serious or valuable ideas by the physicists.
- Problems: there are no sharp problems with this assertion because this assertion is correct, with a sufficiently general definition of the "hair". The known fact that black holes carry a huge entropy really does imply that they have "hair". However, in conventional interpretations, this hair can't be encoded in the configurations of the ordinary low-energy degrees of freedom. Instead, they are encoded in the quantum numbers associated with high-energy fields: see Coleman & Preskill & Wilczek 1992. Now, Samir Mathur's fuzzballs are the closest realization of the picture where the "hair" is encoded in some rather ordinary degrees of freedom but their relevance for generic black holes in quantum gravity remains uncertain. What is certain, however, is that black holes do carry some information and that one needs a very accurate - "quantum accurate" - probes to measure these new degrees of freedom. That's why the low-energy probes and approximations of the full theory should continue to imply that black holes have no (classical) "hair". This condition is satisfied by string theory.
Sabine Hossenfelder wrote seven solutions and incorrectly claimed that all of them have advantages and disadvantages. From reading her general clichés, you have no method to figure out which solution(s) is (are) actually the correct one(s) and why. Her reasons to dislike the correct solution(s) are irrational, as argued below.
In fact, it is fair to say that two "proposed solutions" she listed are correct, namely that the evolution is unitary as known from the AdS/CFT correspondence; and the solution that the black holes do carry some (quantum) "hair", after all. They're both aspects of the same correct solution.
Hossenfelder claimed that every proposed solution has a "disadvantage" so she identifies a "problem" with the correct solutions, too. What's wrong with the solution that the evolution is known to be unitary from the AdS/CFT correspondence? She writes
- Problem: as long as the conjecture is unproved one could equally well consider the information loss problem, if real, as a counter-example for the validity of the conjecture. (Also, I personally would find it unsatisfactory would this only work in AdS space.)
She proposes to assume that the information must be getting lost and this conclusion may even be used to prove that the AdS/CFT correspondence is incorrect. Well, a subtle problem with the approach is that we can see that the AdS/CFT correspondence is valid and every existing argument that the AdS/CFT is invalid or incomplete has a known flaw. On the other hand, every "proof" that the information is lost is known to have a loophole. That's a damn asymmetric situation.
The very existence of the AdS/CFT dual theories is a proof that any "universal" proof that the information must be lost in quantum gravity is incorrect - simply because it is enough to have one counterexample and we have it. ;-) So what she's doing is just complaining that 2+2=4 shouldn't be correct because if she could prove that 1+1=3, it would also mean that 2+2=5. Indeed, one could probably construct such implications but the problem is that the main assumption, namely 1+1=3 (or information loss), cannot be established at a remotely comparable confidence level as the AdS/CFT correspondence. Not only it cannot be established: Hossenfelder's verbal exercise hasn't produced a glimpse of evidence, not even circumstantial or speculative evidence. You shouldn't blame a correct answer for merely being incompatible with the alternative, incorrect answers: that's what correct answers usually "do". ;-)
As far as her personal objection goes, it is ludicrous, too. While the AdS/CFT proof directly applies to anti de Sitter black holes only, the general conclusion that the information is preserved is surely much more general. For example, flat space may be obtained as a limit of a weakly curved AdS space. Moreover, black holes in certain flat spaces can be described in equally unitary Matrix theory, too.
In de Sitter space, the conservation of information is more subtle because even empty de Sitter space seems to have an "unpredictable" thermal radiation coming from the cosmic horizon. There is no "asymptotic region" of a de Sitter space and the information debates become more subtle. But they don't become more subtle because of black holes in the interior of the de Sitter space.
Black holes are localized objects and the behavior of these systems is surely universal: the local processes in string theory exhibit some properties that don't depend on remote regions - string theory is known to satisfy clustering, at least in some mild forms.
The only way how the asymptotic conditions of spacetime influence the conservation of energy is that
- for some of these asymptotic conditions, a new description of the physical system (AdS/CFT or Matrix theory) is known
- the information can only be accurately measured if you have a large enough (or infinite) space where the properties of the Hawking particles may be measured. That's difficult in compact spaces or de Sitter space. But this difficulty is unrelated to the original justification of the black hole paradox.
The AdS/CFT correspondence and Matrix theory are exactly unitary theories consistent with general relativity, the existence of black holes, and their evaporation in the low-energy limit and any proof that such a combination can't exist is wrong. Irreligion has become possible. Religion hasn't quite been proved impossible but it is no longer needed for the most rationally acceptable solution.
If you accept that the AdS black holes preserve the information but you claim that others don't, it's like accepting that Neil Armstrong used a spacecraft to get to the Moon but others will still have to walk. Jesus Christ: if we have already overcome the technical problems and created a spacecraft, why wouldn't we assume that other astronauts can use it, too? Again, I can't rigorously prove that you can't walk to the Moon, using this method. But I can see that the walking becomes a very contrived assumption once I know a gadget - the spacecraft - that can help you (and anyone) to get there.
Event horizons vs singularity: what defines a black hole
But later in her text, Sabine Hossenfelder exhibits her complete incompetence much more explicitly than by hiding the correct answers in the melting pot of five other answers that are known to be extremely unlikely. She also offers her own "idea" that is downright ludicrous. She claims that the essence of the paradox - and the key part of black holes in general - is not in the event horizon but in the singularity.
That's a misunderstanding shared by some undergraduate students who are just beginning to study physics of general relativity and who don't really understand the concepts well. It's analogous to young students of medicine who can't yet recognize stomachs from hearts. In reality, it is not the singularity but the event horizon that defines a black hole and it is the event horizon, not the singularity, that is relevant for the black hole information puzzle.
This statement, completely mysterious to Ms Hossenfelder, is completely elementary. Even the first sentence that defines a black hole at Wikipedia mentions the event horizon as a defining feature of black holes. The light cannot escape from the hole which is why it is (approximately) black. And it cannot escape because of the event horizon, not because of the singularity.
Why is the event horizon, and not the singularity, the crucial place that was responsible for the paradox? Simply because the general relativistic argument relevant for the paradox said that the information cannot escape from the black hole - that's how we know that it is lost. The region that separates the interior and the exterior is the event horizon. Its existence, combined with causality, makes it impossible for the information to get away.
When we talk about the possible information loss, we are comparing two slices through the whole spacetime: one slice occurs before the black hole is formed and another one occurs after the black hole evaporates. None of these slices contains any singularity which is why the singularity cannot be directly relevant for the paradox.
Complicated high-energy processes can take place and do take place near the singularity and the singularity is, most likely, not exactly "singular" when full physics of quantum gravity is taken into account. But that doesn't help us to solve the paradox. The information may be getting lost at the singularity, from the viewpoint of an observer who fell into the black hole and who is going to be destroyed, too.
But this unlucky observer is not the guy who defines the information loss paradox. It is not shocking that a person who is destroyed in a catastrophic gravitational field won't be able to reconstruct the information - because this guy has no optimistic future: in fact, he has no future at all. The information loss paradox arises when the past and future slices of the observer at infinity - outside the black hole - are compared with each other. Whether some unlucky observer inside the black hole thinks that the information was destroyed exactly when he died has no direct relevance for the question whether - and how - the information is lost from the asymptotic observer's viewpoint. The region around the singularity is localized and whatever happens there - and whichever "regularization" of the singularity the full theory chooses - has no impact on the conclusion that the information can't get away.
Summary
The black hole information puzzle is a textbook example of a theorist's puzzle par excellence. It is almost guaranteed that the exact information needed to reconstruct the initial state from the final state of the radiation will never be observed. So far, we haven't even observed the radiation itself. So this discussion has always been theoretical in character and it will probably remain forever theoretical.
But that doesn't mean that correct answers to such questions can't be found. Quite on the contrary, the black hole information puzzle is an example of a question where the correct answer is already known - the information is preserved and the causality is broken by tiny "tunneling" effects that make it possible for the information to escape.
While we know the correct answer, it is often difficult to pinpoint the exact problem of the previous ideas that have led to wrong answers. Why? Because these wrong answers usually assume the existence of degrees of freedom, terms in the action, and other objects that haven't been confirmed in the full theory of quantum gravity as we know it today - a description of a theory that is really equivalent to the phrase "string theory". In other words, these wrong solutions have made all kinds of assumptions that turned out to be incorrect.
In the case of fully defined superselections sectors of string theory - such as the models described by the AdS/CFT correspondence or Matrix theory - we know the states and the in-principle equations that transform the initial state to a final state. In many cases, it is difficult to "localize" the information contained in these states at particular points of space.
But that's just another, related lesson we have learned: although it can be shown that the low-energy approximation of string theory is local, the character of locality becomes subtle in extreme conditions, even in the presence of black holes. We can't really say "where the black hole hair is located" but we're not guaranteed that a good answer to this question exists in the first place. String theory implies the existence of many degrees of freedom but their precise association with a point in space is only guaranteed to exist if we avoid extreme deformations of space such as those that exist in the presence black holes. With black holes, degrees of freedom might be disconnected from particular points in space and if you (artificially) associate them with points in space, the resulting dynamics will be acausal and non-local.
Known and unknown things in physics
I am convinced that all competent quantum gravity physicists agree that the information is preserved in flat space or AdS space even though they may have a feeling that "we are still missing a part of the complete picture". Although you can formulate some arguments in favor of this proposition that don't depend on string theory, it is clear that string theory is by far the most important toolkit that makes us know that the information is preserved. It's also the main reason why Stephen Hawking has joined us to say that the information is preserved, after all.
One more thing to say: this story is a nice example of the huge explanatory power of string theory. While we may be uncertain which vacuum is the correct one - e.g. how many throats our Calabi-Yau space has if we live in one - there are many qualitative conclusions that string theory allows to make unequivocally. The conclusions about the preserved information are an example. They hold in all the 10^{500} vacua that are discussed under the trademark of the "landscape". These conclusions, and many others, are completely universal.
Black holes play a very special role in quantum gravity because they're the only "fundamental" localizable objects that are heavier than the fundamental scale. While the existence of strings and branes depends on the position in the landscape (or configuration space) and they are only useful concepts if some dimensionless moduli are much smaller than one, black holes are universal: they're also the only universal objects that are e.g. produced in all trans-Planckian collisions.
You may see that the people who don't like to study physics of quantum gravity carefully enough get completely lost even in very qualitative questions about physics. You ask them whether the information is lost and they return you 7 possible answers with 1 superficial paragraph per explanation why they dislike it. The explanations under the correct answers are manifestly wrong and many other explanations are confusing. You can simply see that the "quantum gravity theorists" outside the (broader) string theory have no idea what they're talking about.
In real science, it is crucial that one tries to divide the questions into the answered and unanswered ones as sharply and as rationally as possible. If someone can't answer any questions - e.g. not even the qualitative ones related to the information loss puzzle - her or his approach to physics has clearly made no progress. One can't ever be 100% certain but if you have no questions where your certainty clearly exceeds 90% or at least 50%, you have simply chosen a bad approach. You may have asked too ambitious questions and with no quasi-reliable answers, you have nothing to safely build upon.
The people in this bad situation often realize that they have found nothing and compensate this non-existing knowledge by "certainty" about all kinds of other, usually more detailed questions. Unfortunately, this "certainty" is based on pure guesswork and lacks any kind of rational justification. In most cases, the guesses are wrong. That's what guesses usually "do".
The actual cutting-edge status of physics is that many qualitative questions such as the question "is the information preserved?" have been answered while many detailed questions such as "how many throats our compact manifold has?" are uncertain. It is extremely counterproductive to force scientists to feel ashamed that there exist any open questions - such as the vacuum selection issues - because uncertainty and confusion is an inherent part of the scientific process that no scientist should ever be ashamed of. And it is equally counterproductive to force scientists to pretend the uncertainty about questions where they feel certain - such as the qualitative answers to the information loss puzzle.
Scientists should not only "try to know" but they should also "try to know what they know" and "try to know what they don't". It's bad to scream that known things should become unknown and everyone should try to build "diversity" about them, and it's equally bad to scream that unknown things shouldn't exist because a scientist who doesn't know something (or everything) is "not even wrong".
Various people who criticize theoretical physics are making these two complementary mistakes all the time which is too bad.
And that's the memo.
A fun historical paper: Stephen Hawking, Nature 248 (1974), Black hole explosions?
Black hole information puzzle
 Reviewed by DAL
on
June 25, 2008
Rating:
Reviewed by DAL
on
June 25, 2008
Rating:



No comments: